The number 19 is a whole number and can be expressed as a fraction in its simplest form as 19/1.
However, if you're looking for a fraction that's equivalent to 19 but has a denominator other than 1, you can express it as:
19 = 19/1 = 38/2 = 57/3 = 76/4 =...
But in simplest form, 19 is still 19/1.
Let's dive deeper into fractions and explore some concepts related to 19 as a fraction.
What is a Fraction?

A fraction is a way to express a part of a whole. It consists of a numerator (the top number) and a denominator (the bottom number). The numerator tells us how many equal parts we have, and the denominator tells us how many parts the whole is divided into.
For example, the fraction 3/4 means we have 3 equal parts out of a total of 4 parts.
Types of Fractions
There are three main types of fractions:
- Proper fractions: These are fractions where the numerator is less than the denominator. Examples include 1/2, 3/4, and 2/3.
- Improper fractions: These are fractions where the numerator is greater than or equal to the denominator. Examples include 3/2, 5/4, and 7/3.
- Mixed fractions: These are fractions that have a whole number part and a proper fraction part. Examples include 2 1/2, 3 3/4, and 1 1/3.
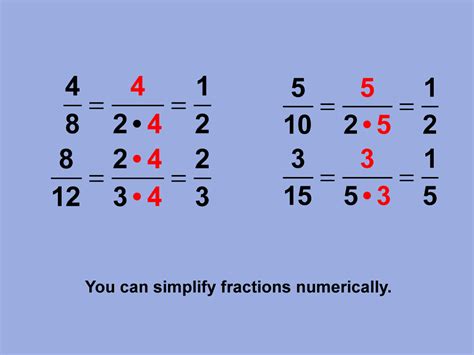
Simplifying Fractions

To simplify a fraction, we need to find the greatest common divisor (GCD) of the numerator and the denominator. The GCD is the largest number that divides both numbers without leaving a remainder.
For example, let's simplify the fraction 6/8:
- Find the GCD of 6 and 8, which is 2.
- Divide both the numerator and the denominator by the GCD: 6 ÷ 2 = 3, 8 ÷ 2 = 4.
- The simplified fraction is 3/4.
Real-World Applications of Fractions
Fractions are used in various real-world applications, such as:
- Cooking: When following a recipe, you may need to use fractions to measure ingredients. For example, 1/4 cup of flour or 3/4 teaspoon of salt.
- Music: Fractions are used in music to represent time signatures and rhythm. For example, 3/4 time or 4/4 time.
- Finance: Fractions are used in finance to calculate interest rates, investment returns, and currency exchange rates.
Converting Fractions to Decimals

To convert a fraction to a decimal, we can divide the numerator by the denominator.
For example, let's convert the fraction 3/4 to a decimal:
- Divide the numerator by the denominator: 3 ÷ 4 = 0.75.
- The decimal equivalent of 3/4 is 0.75.
Converting Decimals to Fractions
To convert a decimal to a fraction, we can use the following steps:
- Write the decimal as a fraction with a denominator of 1: 0.75 = 0.75/1.
- Multiply the numerator and the denominator by 100 to eliminate the decimal: 0.75 × 100 = 75, 1 × 100 = 100.
- Simplify the fraction: 75/100 = 3/4.
Common Fraction Mistakes

Here are some common mistakes people make when working with fractions:
- Adding or subtracting fractions with different denominators without finding a common denominator.
- Multiplying or dividing fractions without inverting and multiplying.
- Simplifying fractions without finding the greatest common divisor.
By avoiding these common mistakes, you can work with fractions confidently and accurately.
Best Practices for Working with Fractions
Here are some best practices for working with fractions:
- Always simplify fractions to their lowest terms.
- Use a common denominator when adding or subtracting fractions.
- Invert and multiply when dividing fractions.
- Check your work to ensure accuracy.
By following these best practices, you can become proficient in working with fractions and apply them to various real-world situations.
In conclusion, fractions are an essential part of mathematics, and understanding them is crucial for problem-solving and critical thinking. By mastering fractions, you can improve your math skills and apply them to various real-world applications.
Now, we'd like to hear from you! What are some common challenges you face when working with fractions? Share your thoughts and experiences in the comments below.
FAQs:
What is a fraction?
+A fraction is a way to express a part of a whole. It consists of a numerator (the top number) and a denominator (the bottom number).
How do I simplify a fraction?
+To simplify a fraction, find the greatest common divisor (GCD) of the numerator and the denominator, and then divide both numbers by the GCD.
What are some real-world applications of fractions?
+Fractions are used in cooking, music, finance, and various other real-world applications.