Graphing Simplified: Slope Intercept Form Made Easy

Quick Links :

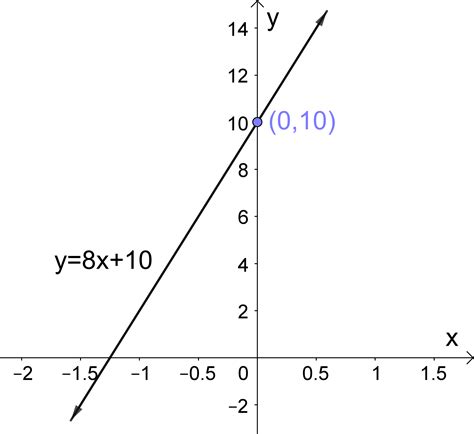
As a fundamental concept in mathematics, graphing is an essential skill for students to master. However, the complexity of graphing can often intimidate young learners, making it challenging for them to grasp the concept. One of the most commonly used forms of graphing is the slope-intercept form, which can seem daunting at first, but with the right approach, it can be made easy to understand and work with. In this article, we will break down the slope-intercept form, providing a comprehensive guide on how to graph with ease.

Understanding Slope Intercept Form

The slope-intercept form is a way of expressing a linear equation in the form y = mx + b, where m represents the slope of the line, and b represents the y-intercept. The slope-intercept form is one of the most commonly used forms of graphing because it provides a clear and concise way of representing linear relationships.

Breaking Down the Slope Intercept Form

To understand the slope-intercept form, it's essential to break down the components of the equation. The slope, represented by the variable m, is a measure of how steep the line is. The slope can be positive, negative, or zero, and it determines the direction of the line. The y-intercept, represented by the variable b, is the point at which the line intersects the y-axis.

Graphing with Slope Intercept Form

Now that we have a clear understanding of the slope-intercept form, let's move on to graphing. Graphing with the slope-intercept form involves two main steps: finding the y-intercept and using the slope to plot additional points.

Step 1: Finding the Y-Intercept

The first step in graphing with the slope-intercept form is to find the y-intercept. The y-intercept is the point at which the line intersects the y-axis, and it's represented by the variable b in the equation. To find the y-intercept, simply substitute the value of b into the equation and plot the point on the graph.

Step 2: Using the Slope to Plot Additional Points

Once you have found the y-intercept, you can use the slope to plot additional points on the graph. The slope represents the change in y for every one-unit change in x. To plot additional points, start at the y-intercept and move up or down by the slope value. For example, if the slope is 2, move up 2 units for every one-unit increase in x.

Examples of Graphing with Slope Intercept Form

Let's take a look at some examples of graphing with the slope-intercept form. Suppose we have the equation y = 2x + 3. To graph this equation, we would first find the y-intercept by substituting the value of b into the equation. In this case, the y-intercept is 3, so we would plot the point (0, 3) on the graph. Next, we would use the slope to plot additional points. Since the slope is 2, we would move up 2 units for every one-unit increase in x.

Common Mistakes to Avoid When Graphing with Slope Intercept Form

While graphing with the slope-intercept form can seem intimidating at first, there are several common mistakes to avoid. One of the most common mistakes is failing to find the y-intercept correctly. Make sure to substitute the value of b into the equation and plot the point on the graph. Another common mistake is using the slope incorrectly. Remember to move up or down by the slope value for every one-unit change in x.

Practical Applications of Slope Intercept Form

The slope-intercept form has numerous practical applications in real-world scenarios. For example, in physics, the slope-intercept form is used to describe the motion of objects. In economics, the slope-intercept form is used to model the relationship between supply and demand. In computer science, the slope-intercept form is used in algorithms for graphing and visualization.

Conclusion: Mastering the Slope Intercept Form

In conclusion, the slope-intercept form is a powerful tool for graphing and modeling linear relationships. By breaking down the components of the equation and following the steps outlined in this article, you can master the slope-intercept form and take your graphing skills to the next level. Remember to find the y-intercept correctly, use the slope to plot additional points, and avoid common mistakes. With practice and patience, you'll become proficient in graphing with the slope-intercept form in no time.

We hope this article has helped you understand the slope-intercept form and how to graph with ease. If you have any questions or comments, please don't hesitate to share them below. We'd love to hear from you!