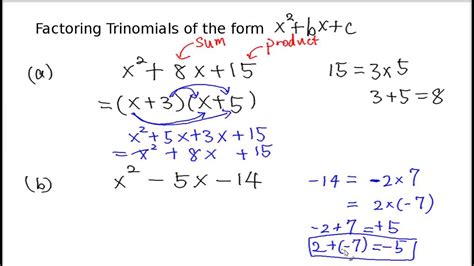
Factoring X2 + 2 is a fundamental concept in algebra that can seem daunting at first, but with the right approach, it can be made easy. In this article, we will break down the concept of factoring X2 + 2, explore its applications, and provide step-by-step instructions on how to factor this type of expression.
What is Factoring X2 + 2?

Factoring X2 + 2 is a mathematical operation that involves expressing the quadratic expression X2 + 2 as a product of two binomials. This process is essential in algebra, as it allows us to simplify complex expressions, solve equations, and graph functions.
Why is Factoring X2 + 2 Important?
Factoring X2 + 2 is crucial in various areas of mathematics and science, including:
- Solving quadratic equations: Factoring X2 + 2 is necessary to solve quadratic equations of the form X2 + 2 = 0.
- Graphing functions: Factoring X2 + 2 helps us to identify the zeros of a quadratic function, which is essential for graphing.
- Simplifying expressions: Factoring X2 + 2 simplifies complex expressions, making it easier to work with them.
How to Factor X2 + 2

Factoring X2 + 2 involves finding two binomials whose product is equal to the original expression. Here are the steps to follow:
- Write the expression: Start by writing the expression X2 + 2.
- Look for two perfect squares: Look for two perfect squares whose sum is equal to 2. In this case, we can use 1 and 1, since 1 + 1 = 2.
- Write the binomials: Write the two binomials as (X + 1) and (X + 1).
- Multiply the binomials: Multiply the two binomials to get the original expression: (X + 1)(X + 1) = X2 + 2.
Example: Factoring X2 + 2
Suppose we want to factor the expression X2 + 2. Using the steps above, we can write:
X2 + 2 = (X + 1)(X + 1)
This shows that X2 + 2 can be factored as the product of two binomials.
Applications of Factoring X2 + 2

Factoring X2 + 2 has numerous applications in mathematics and science, including:
- Solving quadratic equations: Factoring X2 + 2 is necessary to solve quadratic equations of the form X2 + 2 = 0.
- Graphing functions: Factoring X2 + 2 helps us to identify the zeros of a quadratic function, which is essential for graphing.
- Simplifying expressions: Factoring X2 + 2 simplifies complex expressions, making it easier to work with them.
Real-World Examples of Factoring X2 + 2
Factoring X2 + 2 is used in various real-world applications, including:
- Physics: Factoring X2 + 2 is used to solve equations of motion, which describe the motion of objects under the influence of forces.
- Engineering: Factoring X2 + 2 is used to design and optimize systems, such as bridges and electronic circuits.
- Computer Science: Factoring X2 + 2 is used in algorithms for solving quadratic equations and graphing functions.
Common Mistakes to Avoid When Factoring X2 + 2

When factoring X2 + 2, there are several common mistakes to avoid, including:
- Incorrectly identifying the perfect squares: Make sure to identify the correct perfect squares whose sum is equal to 2.
- Incorrectly writing the binomials: Make sure to write the binomials correctly, with the correct signs and coefficients.
- Incorrectly multiplying the binomials: Make sure to multiply the binomials correctly, using the distributive property.
Tips and Tricks for Factoring X2 + 2
Here are some tips and tricks to help you factor X2 + 2:
- Practice, practice, practice: The more you practice factoring X2 + 2, the more comfortable you will become with the process.
- Use online resources: There are many online resources available to help you practice factoring X2 + 2, including video tutorials and interactive exercises.
- Break down the problem: Break down the problem into smaller steps, and focus on one step at a time.
What is the difference between factoring X2 + 2 and factoring X2 - 2?
+The main difference between factoring X2 + 2 and factoring X2 - 2 is the sign of the constant term. When factoring X2 + 2, we look for two perfect squares whose sum is equal to 2, while when factoring X2 - 2, we look for two perfect squares whose difference is equal to 2.
How do I know if an expression can be factored as X2 + 2?
+To determine if an expression can be factored as X2 + 2, look for two perfect squares whose sum is equal to the constant term. If you can find such perfect squares, then the expression can be factored as X2 + 2.
What are some common applications of factoring X2 + 2?
+Factoring X2 + 2 has numerous applications in mathematics and science, including solving quadratic equations, graphing functions, and simplifying expressions.
We hope this article has helped you understand the concept of factoring X2 + 2 and how to apply it in various contexts. With practice and patience, you can become proficient in factoring X2 + 2 and solve complex problems with ease. Share your thoughts and questions in the comments section below, and don't forget to share this article with your friends and classmates who may find it helpful.