Convert Polar To Rectangular Form With Ease Online

Quick Links :

The world of mathematics has always been a fascinating one, filled with complex problems and innovative solutions. One such concept that has been a cornerstone of various mathematical disciplines is the conversion of polar coordinates to rectangular form. This process has numerous applications in fields like engineering, physics, and mathematics itself. In this article, we will delve into the world of polar to rectangular conversion, exploring its importance, the process involved, and the tools available online to make this conversion easier.

Understanding Polar Coordinates

Polar coordinates are a two-dimensional coordinate system where each point on a plane is determined by a distance from a reference point and the angle from a reference direction. This system is particularly useful for describing periodic phenomena and circular motion. However, there are instances where converting these coordinates to rectangular (Cartesian) form is necessary, especially for calculations and graphical representations.

Why Convert Polar to Rectangular Form?

Converting polar coordinates to rectangular form offers several advantages, including ease of computation and visualization. In many mathematical and scientific applications, rectangular coordinates are preferred for their simplicity and the ease with which calculations can be performed. This conversion is especially useful in graphing complex equations and in physics for calculating distances, velocities, and accelerations.

The Conversion Process

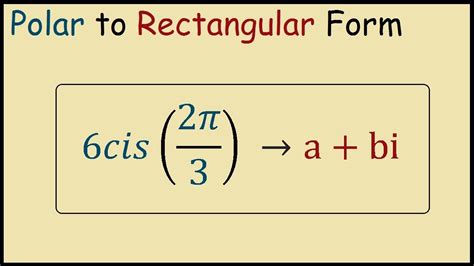
The conversion from polar to rectangular form involves basic trigonometric functions. If we have a point in polar coordinates (r, θ), where 'r' is the radius (distance from the origin) and 'θ' is the angle in radians, we can convert it to rectangular coordinates (x, y) using the following formulas:

• x = r * cos(θ)
• y = r * sin(θ)

These formulas utilize the sine and cosine functions to determine the horizontal (x) and vertical (y) components of the point in rectangular coordinates.

Practical Examples

Let's consider an example to illustrate this conversion:

Given a point in polar coordinates (5, π/4), we can convert it to rectangular form as follows:

• x = 5 * cos(π/4) = 5 * 0.7071 ≈ 3.5355
• y = 5 * sin(π/4) = 5 * 0.7071 ≈ 3.5355

Therefore, the rectangular coordinates of the point are approximately (3.5355, 3.5355).

Online Tools for Conversion

With the advancement of technology and the internet, several online tools and calculators have been developed to facilitate the conversion of polar to rectangular coordinates. These tools are user-friendly, efficient, and provide accurate results, saving time and reducing the risk of human error.

Benefits of Using Online Tools

• Speed and Accuracy: Online tools perform calculations much faster and with greater accuracy compared to manual calculations.
• Simplicity: They offer a simple and intuitive interface, making it easy for users to input polar coordinates and obtain rectangular coordinates instantly.
• Accessibility: Online tools are accessible from anywhere, at any time, as long as there is an internet connection.

Conclusion and Call to Action

The conversion of polar coordinates to rectangular form is a fundamental process in mathematics and science. With the availability of online tools, this process has become more straightforward and accessible. Whether you are a student, researcher, or professional, understanding this conversion and utilizing online resources can significantly enhance your work efficiency and accuracy.

We invite you to explore the world of polar to rectangular conversion further and to share your experiences or tips on using online tools for mathematical conversions. Feel free to comment below and share this article with others who might find it informative and helpful.