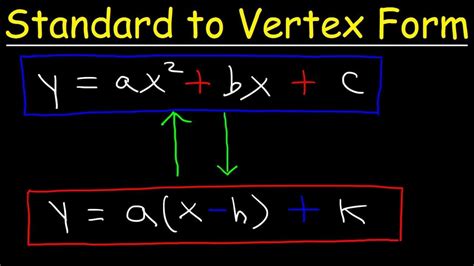
Converting a quadratic equation to vertex form is a crucial skill in algebra, as it allows us to easily identify the vertex of a parabola. The vertex form of a quadratic equation is written as y = a(x - h)^2 + k, where (h, k) is the vertex of the parabola. In this article, we will explore the two easy steps to convert a quadratic equation to vertex form.

Why is Vertex Form Important?
Before we dive into the steps, let's quickly discuss why vertex form is important. The vertex form of a quadratic equation provides valuable information about the parabola, such as the location of the vertex, the axis of symmetry, and the direction of the parabola. This information is essential in graphing parabolas, solving problems in physics and engineering, and modeling real-world situations.
Step 1: Complete the Square
The first step in converting a quadratic equation to vertex form is to complete the square. This involves rewriting the quadratic equation in a form that allows us to easily identify the vertex. To complete the square, we need to move the constant term to the right-hand side of the equation and then group the like terms.
For example, let's consider the quadratic equation y = x^2 + 6x + 8. To complete the square, we can rewrite the equation as:
y = x^2 + 6x + 9 - 1
This allows us to group the like terms and rewrite the equation as:
y = (x + 3)^2 - 1
As we can see, completing the square helps us to rewrite the quadratic equation in a form that is closer to the vertex form.

Step 2: Write in Vertex Form
The second step is to write the quadratic equation in vertex form. This involves identifying the vertex and rewriting the equation in the form y = a(x - h)^2 + k.
Using the example from Step 1, we can identify the vertex as (-3, -1) and rewrite the equation in vertex form as:
y = (x + 3)^2 - 1
This is the vertex form of the quadratic equation, where (h, k) = (-3, -1) is the vertex of the parabola.

Benefits of Vertex Form
The vertex form of a quadratic equation provides several benefits, including:
- Easy identification of the vertex and axis of symmetry
- Simple graphing of parabolas
- Easy solution of problems in physics and engineering
- Efficient modeling of real-world situations

Real-World Applications
The vertex form of a quadratic equation has numerous real-world applications, including:
- Physics: modeling the trajectory of projectiles
- Engineering: designing roller coasters and other thrill rides
- Economics: modeling supply and demand curves
- Computer Science: modeling algorithms and data structures

Conclusion
Converting a quadratic equation to vertex form is a simple two-step process that involves completing the square and writing the equation in vertex form. The vertex form provides valuable information about the parabola and has numerous real-world applications. By mastering the vertex form, you can solve problems in physics, engineering, economics, and computer science with ease.
Call to Action
Now that you know the two easy steps to convert a quadratic equation to vertex form, it's time to practice! Try converting different quadratic equations to vertex form and explore the various real-world applications. Share your experiences and ask questions in the comments below. Don't forget to share this article with your friends and classmates who may benefit from learning about vertex form.
FAQ Section:
What is the vertex form of a quadratic equation?
+The vertex form of a quadratic equation is written as y = a(x - h)^2 + k, where (h, k) is the vertex of the parabola.
Why is completing the square important in converting to vertex form?
+Completing the square helps us to rewrite the quadratic equation in a form that allows us to easily identify the vertex.
What are some real-world applications of vertex form?
+The vertex form has numerous real-world applications, including physics, engineering, economics, and computer science.