Exponential Form Explained In 5 Easy Ways

Quick Links :

Exponential form, also known as exponential notation, is a mathematical shorthand used to express repeated multiplication of a number by itself. It's a fundamental concept in mathematics, science, and engineering, and is used to describe phenomena like population growth, chemical reactions, and electric circuits. In this article, we'll break down the concept of exponential form into 5 easy ways to help you understand and work with it.

What is Exponential Form?

Exponential form is a way of writing numbers that are the result of repeated multiplication of a base number by itself. It's denoted by a base number raised to a power, which is called the exponent. For example, 2^3 means 2 multiplied by itself 3 times: 2 × 2 × 2 = 8.

Why is Exponential Form Important?

Exponential form is essential in mathematics and science because it helps to simplify complex calculations and describes real-world phenomena. It's used in various fields, including finance, biology, physics, and engineering, to model population growth, chemical reactions, electric circuits, and more.

Way 1: Understanding the Basics of Exponential Form

To understand exponential form, you need to know the following:

• The base number: This is the number that is being multiplied by itself.
• The exponent: This is the power to which the base number is raised.
• The result: This is the product of the base number multiplied by itself the number of times indicated by the exponent.

For example, in the expression 2^3, 2 is the base number, 3 is the exponent, and 8 is the result.

Exponential Form Examples

• 2^4 = 2 × 2 × 2 × 2 = 16
• 3^2 = 3 × 3 = 9
• 4^1 = 4

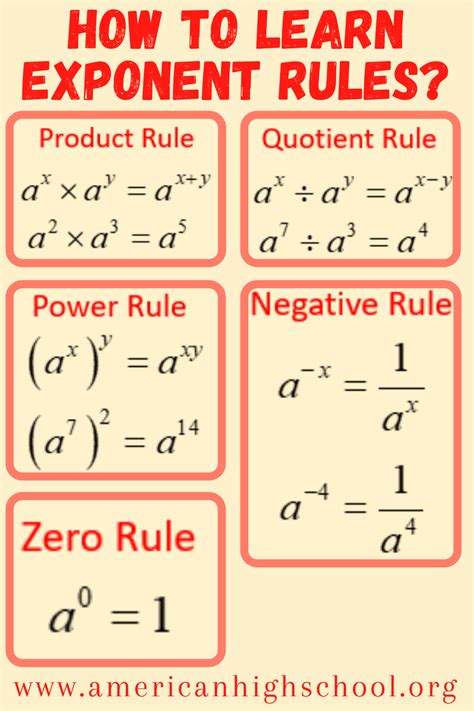
Way 2: Working with Exponents

When working with exponents, you need to follow these rules:

• When multiplying numbers with the same base, add the exponents: 2^2 × 2^3 = 2^(2+3) = 2^5
• When dividing numbers with the same base, subtract the exponents: 2^5 ÷ 2^2 = 2^(5-2) = 2^3
• When raising a number to a power, multiply the exponents: (2^2)^3 = 2^(2×3) = 2^6

Exponent Rules Examples

• 2^2 × 2^3 = 2^5 = 32
• 2^5 ÷ 2^2 = 2^3 = 8
• (2^2)^3 = 2^6 = 64

Way 3: Using Exponential Form in Real-World Applications

Exponential form is used in various real-world applications, including:

• Finance: Compound interest, population growth, and stock market analysis
• Biology: Modeling population growth, disease spread, and chemical reactions
• Physics: Describing electric circuits, sound waves, and light waves
• Engineering: Designing electronic circuits, bridges, and buildings

Real-World Examples

• Compound interest: A = P(1 + r)^n, where A is the amount, P is the principal, r is the interest rate, and n is the number of years
• Population growth: P(t) = P0 * (1 + r)^t, where P(t) is the population at time t, P0 is the initial population, r is the growth rate, and t is the time

Way 4: Simplifying Exponential Expressions

Simplifying exponential expressions involves applying the rules of exponents and factoring out common bases. For example:

• 2^2 × 2^3 = 2^(2+3) = 2^5
• (2^2)^3 = 2^(2×3) = 2^6

Simplification Examples

• 2^2 × 2^3 = 2^5 = 32
• (2^2)^3 = 2^6 = 64
• 3^2 × 3^4 = 3^(2+4) = 3^6 = 729

Way 5: Visualizing Exponential Growth

Visualizing exponential growth involves using graphs and charts to illustrate the rapid increase in a quantity over time. For example:

• A population growing at a rate of 2% per year will double in size every 35 years
• A bank account earning compound interest at a rate of 5% per year will grow exponentially over time

Visualization Examples

• A population growth graph showing the rapid increase in population over time
• A compound interest graph showing the exponential growth of an investment over time

We hope this article has helped you understand the concept of exponential form and its importance in mathematics and science. By following these 5 easy ways, you'll be able to work with exponents, simplify exponential expressions, and visualize exponential growth.

Feel free to share your thoughts, ask questions, or provide feedback on this article. We'd love to hear from you!