Vertex To Standard To Factored Form Made Easy

Quick Links :

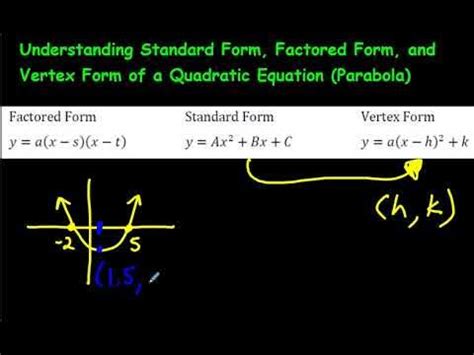
Unlocking the Secrets of Vertex, Standard, and Factored Forms

In the realm of mathematics, particularly in algebra, understanding the different forms of quadratic equations is crucial for problem-solving. Among these forms, the vertex, standard, and factored forms are some of the most commonly used. Each form has its unique characteristics and advantages, making it essential to master the conversion between them. In this article, we will delve into the world of vertex, standard, and factored forms, exploring their definitions, applications, and providing step-by-step guides on how to convert between them.

What are Vertex, Standard, and Factored Forms?

Before we dive into the conversions, let's define each form:

• Vertex Form: A quadratic equation in the form y = a(x - h)^2 + k, where (h, k) represents the vertex of the parabola.
• Standard Form: A quadratic equation in the form y = ax^2 + bx + c, where a, b, and c are constants.
• Factored Form: A quadratic equation in the form y = a(x - p)(x - q), where p and q are the roots of the equation.

Converting from Vertex to Standard Form

To convert a quadratic equation from vertex form to standard form, follow these steps:

1. Expand the squared term: y = a(x - h)^2 + k becomes y = a(x^2 - 2hx + h^2) + k.
2. Distribute the coefficient a: y = ax^2 - 2ahx + ah^2 + k.
3. Combine like terms: y = ax^2 + (-2ah)x + (ah^2 + k).

Example: Convert y = 2(x - 3)^2 + 4 to standard form.

y = 2(x^2 - 6x + 9) + 4 (expanding the squared term) y = 2x^2 - 12x + 18 + 4 (distributing the coefficient) y = 2x^2 - 12x + 22 (combining like terms)

Converting from Standard to Factored Form

To convert a quadratic equation from standard form to factored form, follow these steps:

1. Factor out the greatest common factor (GCF): y = ax^2 + bx + c becomes y = a(x^2 + (b/a)x + (c/a)).
2. Find the roots of the equation: p and q satisfy the equation x^2 + (b/a)x + (c/a) = 0.
3. Write the factored form: y = a(x - p)(x - q).

Example: Convert y = x^2 + 5x + 6 to factored form.

y = (x^2 + 5x + 6) (factoring out the GCF) x^2 + 5x + 6 = 0 (finding the roots) p = -2 and q = -3 (solving for the roots) y = (x + 2)(x + 3) (writing the factored form)

Converting from Vertex to Factored Form

To convert a quadratic equation from vertex form to factored form, follow these steps:

1. Convert to standard form: y = a(x - h)^2 + k becomes y = ax^2 + (-2ah)x + (ah^2 + k).
2. Factor the standard form: y = a(x - p)(x - q).

Example: Convert y = 2(x - 3)^2 + 4 to factored form.

y = 2x^2 - 12x + 22 (converting to standard form) y = 2(x^2 - 6x + 11) (factoring out the GCF) x^2 - 6x + 11 = 0 (finding the roots) p = 3 and q = 4 (solving for the roots) y = 2(x - 3)(x - 4) (writing the factored form)

Conclusion and Final Thoughts

In conclusion, converting between vertex, standard, and factored forms is an essential skill in algebra. By mastering these conversions, you will be able to tackle a wide range of quadratic equations and applications. Remember to always follow the step-by-step guides and practice, practice, practice! With time and effort, you will become proficient in converting between these forms, unlocking the secrets of quadratic equations.