5 Ways To Express Equations In Function Form

Quick Links :

Understanding the Importance of Equations in Function Form

In mathematics, equations are a fundamental concept used to describe relationships between variables. Equations can be expressed in various forms, including function form, which is a crucial concept in algebra and calculus. Expressing equations in function form allows us to analyze and solve problems more efficiently. In this article, we will explore five ways to express equations in function form, highlighting the benefits and applications of each method.

What are Equations in Function Form?

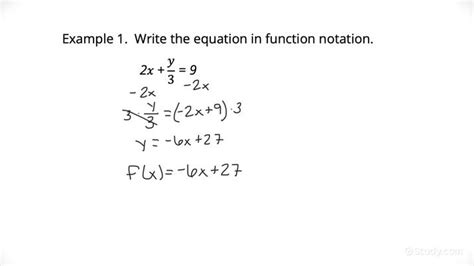
Equations in function form are a way of expressing relationships between variables in a specific format. In this format, the equation is written as f(x) = expression, where f(x) represents the output or result, and x represents the input or variable. The expression on the right-hand side of the equation describes the relationship between the input and output.

Method 1: Using the Slope-Intercept Form

One common method for expressing equations in function form is using the slope-intercept form, which is y = mx + b. In this form, m represents the slope of the line, and b represents the y-intercept. To express an equation in function form using the slope-intercept form, we need to rewrite the equation in the format f(x) = mx + b.

For example, consider the equation 2x + 3y = 5. To express this equation in function form, we can rewrite it as y = (-2/3)x + 5/3. In this example, the slope (m) is -2/3, and the y-intercept (b) is 5/3.

Benefits of Using the Slope-Intercept Form

Using the slope-intercept form to express equations in function form has several benefits. Firstly, it allows us to easily identify the slope and y-intercept of the line, which are crucial in graphing and analyzing linear relationships. Secondly, it provides a straightforward way to rewrite equations in function form, making it easier to solve problems.

Method 2: Using the Point-Slope Form

Another method for expressing equations in function form is using the point-slope form, which is y - y1 = m(x - x1). In this form, (x1, y1) represents a point on the line, and m represents the slope. To express an equation in function form using the point-slope form, we need to rewrite the equation in the format f(x) = m(x - x1) + y1.

For example, consider the equation 2x - 3y = -5. To express this equation in function form, we can rewrite it as y = (2/3)x + 5/3. In this example, the slope (m) is 2/3, and the point (x1, y1) is (0, 5/3).

Benefits of Using the Point-Slope Form

Using the point-slope form to express equations in function form has several benefits. Firstly, it allows us to use a specific point on the line to find the equation of the line. Secondly, it provides a flexible way to rewrite equations in function form, especially when the slope and a point on the line are given.

Method 3: Using the Standard Form

The standard form, also known as the general form, is another method for expressing equations in function form. The standard form is ax + by = c, where a, b, and c are constants. To express an equation in function form using the standard form, we need to rewrite the equation in the format f(x) = (c - ax)/b.

For example, consider the equation 2x + 3y = 5. To express this equation in function form, we can rewrite it as y = (-2/3)x + 5/3. In this example, a = 2, b = 3, and c = 5.

Benefits of Using the Standard Form

Using the standard form to express equations in function form has several benefits. Firstly, it provides a general format for expressing linear equations. Secondly, it allows us to easily identify the coefficients of the variables, which are crucial in solving systems of equations.

Method 4: Using the Vertex Form

The vertex form is a method for expressing quadratic equations in function form. The vertex form is f(x) = a(x - h)^2 + k, where (h, k) represents the vertex of the parabola. To express a quadratic equation in function form using the vertex form, we need to rewrite the equation in the format f(x) = a(x - h)^2 + k.

For example, consider the equation x^2 + 4x + 4 = 0. To express this equation in function form, we can rewrite it as f(x) = (x + 2)^2. In this example, the vertex (h, k) is (-2, 0).

Benefits of Using the Vertex Form

Using the vertex form to express quadratic equations in function form has several benefits. Firstly, it allows us to easily identify the vertex of the parabola, which is crucial in graphing and analyzing quadratic relationships. Secondly, it provides a straightforward way to rewrite quadratic equations in function form, making it easier to solve problems.

Method 5: Using the Factored Form

The factored form is a method for expressing quadratic equations in function form. The factored form is f(x) = a(x - r1)(x - r2), where r1 and r2 represent the roots of the quadratic equation. To express a quadratic equation in function form using the factored form, we need to rewrite the equation in the format f(x) = a(x - r1)(x - r2).

For example, consider the equation x^2 + 5x + 6 = 0. To express this equation in function form, we can rewrite it as f(x) = (x + 2)(x + 3). In this example, the roots (r1 and r2) are -2 and -3.

Benefits of Using the Factored Form

Using the factored form to express quadratic equations in function form has several benefits. Firstly, it allows us to easily identify the roots of the quadratic equation, which are crucial in solving problems. Secondly, it provides a straightforward way to rewrite quadratic equations in function form, making it easier to analyze and solve problems.

Conclusion and Next Steps

In conclusion, expressing equations in function form is a crucial concept in mathematics, and there are various methods to do so. The five methods discussed in this article, including the slope-intercept form, point-slope form, standard form, vertex form, and factored form, provide a range of options for expressing equations in function form. Each method has its benefits and applications, and understanding these methods can help you become more proficient in mathematics.

If you have any questions or need further clarification on any of the methods discussed in this article, please feel free to comment below. Additionally, if you would like to learn more about expressing equations in function form or other mathematical concepts, please let us know, and we will be happy to provide more resources and support.