Slope Intercept To Function Notation Made Easy

Quick Links :

Learning about slope-intercept form and function notation can be a daunting task for many students, but with the right approach, it can be made easy and even enjoyable. In this article, we will break down the concepts of slope-intercept form and function notation, and provide practical examples to help you understand and apply them with confidence.

Understanding Slope-Intercept Form

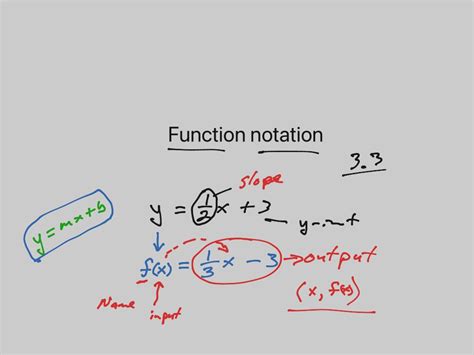
Slope-intercept form is a way of expressing a linear equation in the form y = mx + b, where m is the slope and b is the y-intercept. This form is particularly useful for graphing lines and understanding the relationship between the x and y variables.

To understand slope-intercept form, you need to know what the slope (m) and y-intercept (b) represent. The slope tells you how steep the line is, while the y-intercept tells you where the line crosses the y-axis.

What is Slope?

The slope of a line is a measure of how steep it is. It can be calculated using the formula m = (y2 - y1) / (x2 - x1), where (x1, y1) and (x2, y2) are two points on the line. A positive slope indicates that the line slopes upward from left to right, while a negative slope indicates that the line slopes downward from left to right.

What is Y-Intercept?

The y-intercept is the point where the line crosses the y-axis. It is the value of y when x is equal to 0. The y-intercept can be positive, negative, or zero, depending on the equation of the line.

Understanding Function Notation

Function notation is a way of expressing a relationship between a variable and the output of a function. It is often used to represent a function in a compact and concise way.

In function notation, the input variable is represented by x, and the output variable is represented by f(x). The function notation f(x) = y tells you that the output y is a function of the input x.

What is a Function?

A function is a relation between a set of inputs (called the domain) and a set of possible outputs (called the range). It assigns to each input a unique output.

In mathematics, a function is often represented by a formula or equation that describes the relationship between the input and output variables.

Converting Slope-Intercept Form to Function Notation

Converting slope-intercept form to function notation is a straightforward process. You simply replace the y variable with f(x) and keep the rest of the equation the same.

For example, the slope-intercept equation y = 2x + 3 can be rewritten in function notation as f(x) = 2x + 3.

Examples of Converting Slope-Intercept Form to Function Notation

Here are a few more examples:

• y = 4x - 2 becomes f(x) = 4x - 2
• y = -3x + 5 becomes f(x) = -3x + 5
• y = x/2 + 1 becomes f(x) = x/2 + 1

Benefits of Using Function Notation

Using function notation has several benefits, including:

• It provides a compact and concise way of representing a function.
• It allows you to easily evaluate the function at specific input values.
• It makes it easy to compose functions and create new functions.
• It provides a clear and unambiguous way of communicating mathematical ideas.

Practical Applications of Function Notation

Function notation has many practical applications in science, engineering, economics, and other fields. It is used to model real-world phenomena, such as population growth, financial transactions, and physical systems.

For example, a company might use function notation to model its revenue as a function of the number of units sold. The function notation f(x) = 2x + 500 might represent the company's revenue, where x is the number of units sold.

Common Mistakes to Avoid

When working with slope-intercept form and function notation, there are several common mistakes to avoid:

• Forgetting to replace the y variable with f(x) when converting to function notation.
• Misidentifying the slope and y-intercept in a slope-intercept equation.
• Failing to evaluate the function at specific input values.
• Misusing function notation to represent non-functional relationships.

Tips for Mastering Slope-Intercept Form and Function Notation

Here are a few tips for mastering slope-intercept form and function notation:

• Practice, practice, practice! The more you practice working with slope-intercept form and function notation, the more comfortable you will become.
• Use online resources and video tutorials to supplement your learning.
• Join a study group or find a study buddy to help you stay motivated and accountable.
• Apply what you learn to real-world problems and scenarios.

In conclusion, slope-intercept form and function notation are essential concepts in mathematics that can be made easy with practice and persistence. By understanding the basics of slope-intercept form and function notation, you can build a strong foundation for future math courses and real-world applications.

We hope this article has been informative and helpful. If you have any questions or comments, please don't hesitate to reach out. Share this article with your friends and classmates who may benefit from it.