Solving Quadratic Equations In Fraction Form Made Easy

Quick Links :

Solving quadratic equations can be a daunting task, especially when they are presented in fraction form. However, with the right approach and techniques, solving these types of equations can be made much easier. In this article, we will explore the steps and methods to solve quadratic equations in fraction form, making it more accessible and manageable for students and math enthusiasts alike.

Quadratic equations are a fundamental concept in algebra, and they are used to model a wide range of real-world problems. A quadratic equation is a polynomial equation of degree two, which means the highest power of the variable is two. In general, a quadratic equation can be written in the form ax^2 + bx + c = 0, where a, b, and c are constants.

Understanding Quadratic Equations in Fraction Form

Quadratic equations in fraction form are written as (ax^2 + bx + c) / d = 0, where d is a non-zero constant. This type of equation is often encountered in algebra and calculus, and it can be challenging to solve due to the presence of fractions. However, with the right techniques and methods, solving quadratic equations in fraction form can be made much easier.

Step 1: Simplify the Equation

The first step to solve a quadratic equation in fraction form is to simplify the equation by getting rid of the fraction. This can be done by multiplying both sides of the equation by the denominator, which is d.

For example, consider the equation (2x^2 + 3x + 1) / 4 = 0. To simplify this equation, we can multiply both sides by 4, which gives us 2x^2 + 3x + 1 = 0.

Methods for Solving Quadratic Equations

There are several methods for solving quadratic equations, including factoring, quadratic formula, and completing the square.

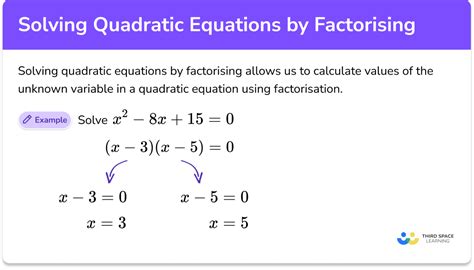
Factoring Method

The factoring method involves expressing the quadratic equation as a product of two binomials. This method is useful when the quadratic equation can be factored easily.

For example, consider the equation x^2 + 5x + 6 = 0. This equation can be factored as (x + 3)(x + 2) = 0.

Quadratic Formula Method

The quadratic formula method involves using the quadratic formula to solve the equation. The quadratic formula is x = (-b ± √(b^2 - 4ac)) / 2a.

For example, consider the equation x^2 + 4x + 4 = 0. Using the quadratic formula, we get x = (-4 ± √(4^2 - 4(1)(4))) / 2(1), which simplifies to x = -2.

Completing the Square Method

The completing the square method involves rewriting the quadratic equation in a perfect square form.

For example, consider the equation x^2 + 6x + 8 = 0. To complete the square, we can rewrite the equation as x^2 + 6x + 9 = 1, which can be factored as (x + 3)^2 = 1.

Practical Applications of Quadratic Equations

Quadratic equations have many practical applications in various fields, including physics, engineering, and economics.

For example, consider a projectile motion problem, where an object is thrown upwards with an initial velocity. The height of the object can be modeled using a quadratic equation, which can be solved to find the maximum height reached by the object.

Conclusion and Final Thoughts

Solving quadratic equations in fraction form can be a challenging task, but with the right approach and techniques, it can be made much easier. By simplifying the equation, using the factoring method, quadratic formula method, or completing the square method, and applying practical applications, students and math enthusiasts can develop a deeper understanding of quadratic equations.

We hope this article has provided valuable insights and information on solving quadratic equations in fraction form. Whether you are a student or a math enthusiast, we encourage you to practice and apply the methods and techniques discussed in this article to improve your skills and knowledge.