5 Ways To Master Writing Equations In Point Slope Form

Quick Links :

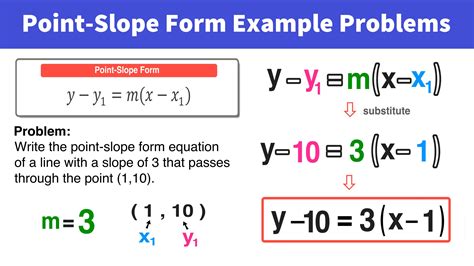
Writing equations in point-slope form is an essential skill for any student of mathematics, particularly those studying algebra and geometry. Mastering this skill can help you solve a wide range of problems, from finding the equation of a line that passes through a given point to determining the slope of a line. In this article, we will explore five ways to master writing equations in point-slope form.

Understanding the Basics of Point-Slope Form

Before we dive into the five ways to master writing equations in point-slope form, let's first review the basics. The point-slope form of a linear equation is given by:

y - y1 = m(x - x1)

where (x1, y1) is a point on the line, and m is the slope of the line. The point-slope form is particularly useful when you know the slope of a line and a point on the line.

Method 1: Using the Slope-Intercept Form

One way to master writing equations in point-slope form is to use the slope-intercept form. The slope-intercept form of a linear equation is given by:

y = mx + b

where m is the slope of the line, and b is the y-intercept. To convert the slope-intercept form to point-slope form, you need to know the slope of the line and a point on the line. Let's say you know the slope (m) and the y-intercept (b), you can choose any point on the line, for example, (0, b). Then, you can write the equation in point-slope form as:

y - b = m(x - 0)

This method is particularly useful when you are given the slope and y-intercept of a line.

Example 1

Find the equation of the line that passes through the point (2, 3) and has a slope of 2.

Solution:

Using the slope-intercept form, we can write the equation as:

y = 2x + b

We can find the value of b by substituting the point (2, 3) into the equation:

3 = 2(2) + b 3 = 4 + b b = -1

Now we can write the equation in point-slope form as:

y - 3 = 2(x - 2)

Method 2: Using the Standard Form

Another way to master writing equations in point-slope form is to use the standard form. The standard form of a linear equation is given by:

Ax + By = C

where A, B, and C are constants. To convert the standard form to point-slope form, you need to know the slope of the line and a point on the line. Let's say you know the standard form of the equation, you can find the slope by dividing both sides of the equation by -B, then dividing by -A. Let's say you find the slope (m) and a point on the line (x1, y1), you can write the equation in point-slope form as:

y - y1 = m(x - x1)

This method is particularly useful when you are given the standard form of a linear equation.

Example 2

Find the equation of the line that passes through the point (1, 2) and has the equation 2x + 3y = 5 in standard form.

Solution:

First, we need to find the slope of the line. We can do this by dividing both sides of the equation by -3:

3y = -2x + 5 y = (-2/3)x + 5/3

The slope of the line is -2/3. Now we can write the equation in point-slope form as:

y - 2 = (-2/3)(x - 1)

Method 3: Using Two Points

A third way to master writing equations in point-slope form is to use two points. If you know two points on a line, you can find the slope of the line and write the equation in point-slope form. Let's say you know two points (x1, y1) and (x2, y2), you can find the slope of the line as:

m = (y2 - y1) / (x2 - x1)

Then, you can write the equation in point-slope form as:

y - y1 = m(x - x1)

This method is particularly useful when you are given two points on a line.

Example 3

Find the equation of the line that passes through the points (2, 3) and (4, 5).

Solution:

First, we need to find the slope of the line:

m = (5 - 3) / (4 - 2) m = 2 / 2 m = 1

Now we can write the equation in point-slope form as:

y - 3 = 1(x - 2)

Method 4: Using the Slope and a Point

A fourth way to master writing equations in point-slope form is to use the slope and a point. If you know the slope of a line and a point on the line, you can write the equation in point-slope form. Let's say you know the slope (m) and a point (x1, y1), you can write the equation in point-slope form as:

y - y1 = m(x - x1)

This method is particularly useful when you are given the slope and a point on a line.

Example 4

Find the equation of the line that has a slope of 3 and passes through the point (1, 2).

Solution:

We can write the equation in point-slope form as:

y - 2 = 3(x - 1)

Method 5: Using a Graph

A fifth way to master writing equations in point-slope form is to use a graph. If you are given a graph of a line, you can find the slope of the line and a point on the line, then write the equation in point-slope form. Let's say you know the graph of a line, you can find the slope of the line by counting the rise over run, then find a point on the line. Let's say you find the slope (m) and a point (x1, y1), you can write the equation in point-slope form as:

y - y1 = m(x - x1)

This method is particularly useful when you are given a graph of a line.

Example 5

Find the equation of the line that passes through the point (2, 3) and has a graph that rises 2 units for every 1 unit it runs.

Solution:

The slope of the line is 2. We can write the equation in point-slope form as:

y - 3 = 2(x - 2)

In conclusion, mastering writing equations in point-slope form requires practice and patience. By using the five methods outlined in this article, you can become proficient in writing equations in point-slope form. Remember to always check your work and use different methods to verify your answers. With time and practice, you will become more confident and proficient in your ability to write equations in point-slope form.

What are your favorite methods for writing equations in point-slope form? Share your thoughts and experiences in the comments below.