1 Metric Unit For Momentum You Need To Know

Quick Links :

Momentum is a fundamental concept in physics that describes the tendency of an object to keep moving in a straight line. It is a measure of the amount of motion an object has, and it depends on two main factors: the object's mass and velocity. Understanding momentum is crucial in various fields, including engineering, physics, and even everyday life. In this article, we will delve into the metric unit for momentum and explore its significance in different contexts.

What is Momentum?

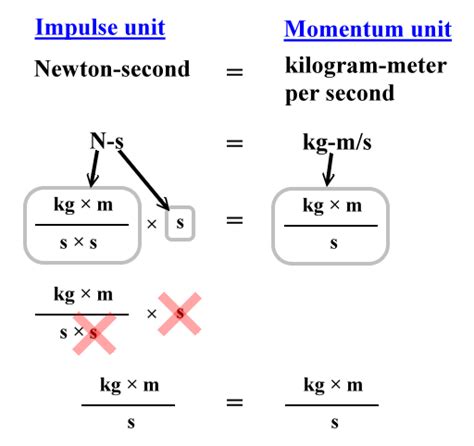
Momentum is a vector quantity, which means it has both magnitude and direction. It is calculated by multiplying an object's mass (in kilograms) by its velocity (in meters per second). The unit of momentum is kilogram-meters per second (kg·m/s). Momentum is often represented by the symbol p and is measured in various units, including:

• kg·m/s (kilogram-meters per second)
• N·s (newton-seconds)
• slug·ft/s (slug-feet per second)

In the International System of Units (SI), the preferred unit of momentum is kg·m/s.

Importance of Momentum in Physics

Momentum plays a vital role in understanding various physical phenomena, such as:

• Collisions: Momentum is conserved in closed systems, meaning that the total momentum before a collision is equal to the total momentum after the collision.
• Rotational motion: Momentum is crucial in describing the motion of rotating objects, such as spinning tops or merry-go-rounds.
• Energy transfer: Momentum is involved in the transfer of energy between objects, such as in the case of a billiard ball hitting another ball.

Metric Unit for Momentum: Kilogram-Meters Per Second (kg·m/s)

The kilogram-meters per second (kg·m/s) is the most commonly used unit for momentum in the metric system. This unit is derived from the product of an object's mass (in kilograms) and velocity (in meters per second).

To calculate momentum in kg·m/s, you can use the following formula:

p = m × v

where p is the momentum, m is the mass in kilograms, and v is the velocity in meters per second.

Examples of Momentum Calculations

1. A car with a mass of 1500 kg is traveling at a velocity of 25 m/s. What is its momentum?

p = 1500 kg × 25 m/s = 37,500 kg·m/s

2. A tennis ball with a mass of 0.057 kg is moving at a velocity of 20 m/s. What is its momentum?

p = 0.057 kg × 20 m/s = 1.14 kg·m/s

Practical Applications of Momentum

Momentum has numerous practical applications in various fields, including:

• Transportation: Understanding momentum is essential in designing and optimizing transportation systems, such as cars, trains, and airplanes.
• Sports: Momentum is crucial in sports, such as tennis, baseball, and football, where players need to understand how to transfer momentum to achieve maximum performance.
• Engineering: Momentum is used in designing and analyzing mechanical systems, such as gears, levers, and pulleys.

Real-World Examples of Momentum

1. A bullet fired from a gun has a high momentum due to its small mass and high velocity.
2. A bowling ball has a large momentum due to its mass and velocity, which allows it to knock down pins.
3. A speeding car has a high momentum, which makes it difficult to stop quickly.

Conclusion: The Significance of Momentum

In conclusion, momentum is a fundamental concept in physics that plays a vital role in understanding various physical phenomena. The metric unit for momentum, kilogram-meters per second (kg·m/s), is widely used in scientific and engineering applications. By understanding momentum, we can analyze and optimize systems, predict outcomes, and make informed decisions.

We encourage you to share your thoughts and examples of momentum in the comments below. How do you apply the concept of momentum in your daily life or work?