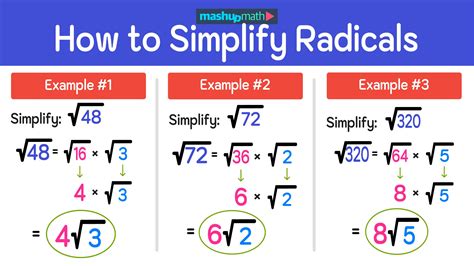
Radicals can be intimidating, especially when they're complex and involve multiple terms. However, with a few simple techniques, you can simplify radicals and make them more manageable. In this article, we'll explore five ways to simplify radicals, along with examples and practical tips to help you master these techniques.
Understanding Radicals
Before we dive into simplifying radicals, it's essential to understand what radicals are and how they work. A radical is a mathematical expression that contains a root, such as a square root or cube root. Radicals are used to represent numbers that cannot be expressed as simple fractions or integers.

Method 1: Factoring Out Perfect Squares
One of the simplest ways to simplify radicals is to factor out perfect squares. A perfect square is a number that can be expressed as the square of an integer, such as 4, 9, or 16. When you see a radical with a perfect square, you can simplify it by factoring out the square root of the perfect square.
For example, let's simplify the radical √(16x^2). Since 16 is a perfect square (4^2), we can factor it out of the radical:
√(16x^2) = √(4^2x^2) = 4√(x^2) = 4x

Example: Simplifying Radicals with Perfect Squares
Simplify the radical √(25x^4).
Since 25 is a perfect square (5^2), we can factor it out of the radical:
√(25x^4) = √(5^2x^4) = 5√(x^4) = 5x^2
Method 2: Using the Product Rule
Another way to simplify radicals is to use the product rule. The product rule states that the product of two radicals is equal to the radical of the product. This means that you can combine two radicals into a single radical.
For example, let's simplify the expression √(2x) √(3y). Using the product rule, we can combine the two radicals:
√(2x) √(3y) = √(2x × 3y) = √(6xy)

Example: Simplifying Radicals with the Product Rule
Simplify the expression √(4x) √(9y).
Using the product rule, we can combine the two radicals:
√(4x) √(9y) = √(4x × 9y) = √(36xy) = 6√(xy)
Method 3: Using the Quotient Rule
The quotient rule is similar to the product rule, but it applies to division instead of multiplication. The quotient rule states that the quotient of two radicals is equal to the radical of the quotient.
For example, let's simplify the expression √(12x) / √(4y). Using the quotient rule, we can simplify the expression:
√(12x) / √(4y) = √(12x / 4y) = √(3x / y)

Example: Simplifying Radicals with the Quotient Rule
Simplify the expression √(18x) / √(9y).
Using the quotient rule, we can simplify the expression:
√(18x) / √(9y) = √(18x / 9y) = √(2x / y)
Method 4: Rationalizing the Denominator
When you have a radical in the denominator of a fraction, it's often helpful to rationalize the denominator. Rationalizing the denominator means multiplying the numerator and denominator by a value that will eliminate the radical in the denominator.
For example, let's simplify the expression 1 / √(2). To rationalize the denominator, we can multiply the numerator and denominator by √(2):
1 / √(2) = (√(2) / √(2)) / (√(2) / √(2)) = √(2) / 2

Example: Rationalizing the Denominator
Simplify the expression 1 / √(3).
To rationalize the denominator, we can multiply the numerator and denominator by √(3):
1 / √(3) = (√(3) / √(3)) / (√(3) / √(3)) = √(3) / 3
Method 5: Using Conjugates
Finally, you can use conjugates to simplify radicals. A conjugate is a value that, when multiplied by the original expression, eliminates the radical.
For example, let's simplify the expression √(2x) + √(3y). To use conjugates, we can multiply the expression by its conjugate:
(√(2x) + √(3y)) × (√(2x) - √(3y)) = (√(2x))^2 - (√(3y))^2 = 2x - 3y

Example: Using Conjugates
Simplify the expression √(4x) + √(9y).
To use conjugates, we can multiply the expression by its conjugate:
(√(4x) + √(9y)) × (√(4x) - √(9y)) = (√(4x))^2 - (√(9y))^2 = 4x - 9y
In conclusion, simplifying radicals is an essential skill in mathematics, and there are several techniques you can use to simplify radicals. By mastering these techniques, you'll be able to tackle even the most complex radical expressions with confidence.
Now that you've learned these techniques, we encourage you to practice simplifying radicals on your own. Try working through some examples, and don't be afraid to ask for help if you get stuck.
What is a radical in mathematics?
+A radical is a mathematical expression that contains a root, such as a square root or cube root.
How do I simplify a radical expression?
+There are several techniques you can use to simplify radical expressions, including factoring out perfect squares, using the product rule and quotient rule, rationalizing the denominator, and using conjugates.
What is the difference between a perfect square and a radical?
+A perfect square is a number that can be expressed as the square of an integer, such as 4 or 9. A radical is a mathematical expression that contains a root, such as a square root or cube root.