Finding intercepts from standard form can be a daunting task for many students, but with the right approach, it can be made easy. In this article, we will explore the concept of intercepts, the standard form of a linear equation, and provide a step-by-step guide on how to find intercepts from standard form.
Understanding Intercepts and Standard Form

To begin with, let's define what intercepts are. In the context of linear equations, intercepts refer to the points at which the graph of the equation crosses the x-axis or the y-axis. The x-intercept is the point where the graph crosses the x-axis, while the y-intercept is the point where the graph crosses the y-axis.
The standard form of a linear equation is given by the formula: Ax + By = C, where A, B, and C are constants. In this form, the coefficients A and B represent the slope and the y-intercept of the line, respectively.
Why Finding Intercepts is Important
Finding intercepts is crucial in understanding the behavior of linear equations. By determining the x-intercept and y-intercept, we can identify the points at which the graph crosses the axes, which provides valuable information about the equation.
For instance, in real-world applications, intercepts can be used to model situations such as the cost of production, the rate of change of a physical quantity, or the position of an object in space. By finding the intercepts, we can gain insight into the underlying relationships between variables.
Step-by-Step Guide to Finding Intercepts from Standard Form

Now that we have covered the basics, let's dive into the step-by-step guide on how to find intercepts from standard form.
Step 1: Identify the Standard Form of the Equation
The first step is to ensure that the equation is in standard form, which is Ax + By = C. If the equation is not in standard form, we need to rearrange it to obtain the standard form.
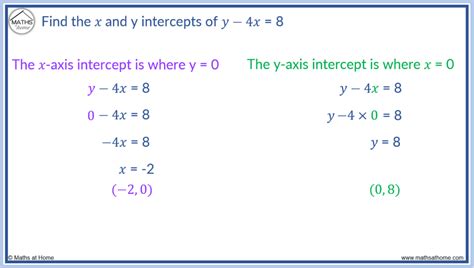
Step 2: Find the X-Intercept
To find the x-intercept, we set y = 0 and solve for x. This is because the x-intercept occurs when the graph crosses the x-axis, which is when y = 0.
For example, consider the equation 2x + 3y = 6. To find the x-intercept, we set y = 0 and solve for x:
2x + 3(0) = 6 2x = 6 x = 3
Therefore, the x-intercept is (3, 0).
Step 3: Find the Y-Intercept
To find the y-intercept, we set x = 0 and solve for y. This is because the y-intercept occurs when the graph crosses the y-axis, which is when x = 0.
Using the same example, we set x = 0 and solve for y:
2(0) + 3y = 6 3y = 6 y = 2
Therefore, the y-intercept is (0, 2).
Practical Examples and Statistical Data

To illustrate the concept of finding intercepts from standard form, let's consider some practical examples and statistical data.
Example 1: A company's cost of production is given by the equation 2x + 5y = 100, where x is the number of units produced and y is the cost per unit. Find the x-intercept and y-intercept.
Solution: To find the x-intercept, we set y = 0 and solve for x:
2x + 5(0) = 100 2x = 100 x = 50
Therefore, the x-intercept is (50, 0), which means that the company can produce 50 units at a cost of $0 per unit.
To find the y-intercept, we set x = 0 and solve for y:
2(0) + 5y = 100 5y = 100 y = 20
Therefore, the y-intercept is (0, 20), which means that the company's fixed cost is $20.
Example 2: A study found that the relationship between the amount of exercise and the risk of heart disease is given by the equation x - 2y = 5, where x is the amount of exercise and y is the risk of heart disease. Find the x-intercept and y-intercept.
Solution: To find the x-intercept, we set y = 0 and solve for x:
x - 2(0) = 5 x = 5
Therefore, the x-intercept is (5, 0), which means that exercising for 5 hours reduces the risk of heart disease to 0.
To find the y-intercept, we set x = 0 and solve for y:
0 - 2y = 5 -2y = 5 y = -2.5
Therefore, the y-intercept is (0, -2.5), which means that not exercising increases the risk of heart disease by 2.5 times.
Benefits of Finding Intercepts

Finding intercepts from standard form provides numerous benefits, including:
- Understanding the behavior of linear equations
- Identifying the points at which the graph crosses the axes
- Modeling real-world situations, such as cost of production, rate of change, and position of an object in space
- Making informed decisions based on statistical data
In conclusion, finding intercepts from standard form is a crucial aspect of linear equations. By following the step-by-step guide and understanding the benefits of finding intercepts, you can gain a deeper insight into the behavior of linear equations and make informed decisions based on statistical data.
Take Action

Now that you have learned how to find intercepts from standard form, it's time to put your knowledge into practice. Take a few minutes to try out some examples and see how finding intercepts can help you understand the behavior of linear equations.
Share your thoughts and experiences in the comments section below. How do you find intercepts from standard form? What are some practical applications of finding intercepts?
FAQ Section:
What is the standard form of a linear equation?
+The standard form of a linear equation is given by the formula: Ax + By = C, where A, B, and C are constants.
Why is finding intercepts important?
+Finding intercepts is important because it helps us understand the behavior of linear equations, identify the points at which the graph crosses the axes, and model real-world situations.
How do I find the x-intercept from standard form?
+To find the x-intercept, set y = 0 and solve for x.