Convert Intercept Form To Standard Form Easily Today

Quick Links :

Unlock the Power of Linear Equations: A Comprehensive Guide to Converting Intercept Form to Standard Form

Linear equations are a fundamental concept in mathematics, and understanding how to convert between different forms is crucial for problem-solving and analytical thinking. In this article, we will delve into the world of linear equations, focusing on the conversion from intercept form to standard form. By the end of this guide, you will be equipped with the knowledge and skills to tackle even the most complex linear equations with confidence.

The Importance of Linear Equations in Real-Life Applications

Linear equations have numerous practical applications in various fields, including physics, engineering, economics, and computer science. They are used to model real-world phenomena, make predictions, and optimize solutions. For instance, linear equations can be used to:

• Describe the motion of objects in physics
• Model population growth in biology
• Analyze market trends in economics
• Optimize network flow in computer science

Understanding Intercept Form and Standard Form

Before diving into the conversion process, let's review the basics of intercept form and standard form.

Intercept Form:

The intercept form of a linear equation is written as:

y = mx + b

where:

• y is the dependent variable
• x is the independent variable
• m is the slope of the line
• b is the y-intercept (the point where the line crosses the y-axis)

Standard Form:

The standard form of a linear equation is written as:

Ax + By = C

where:

• A, B, and C are constants
• x and y are the variables

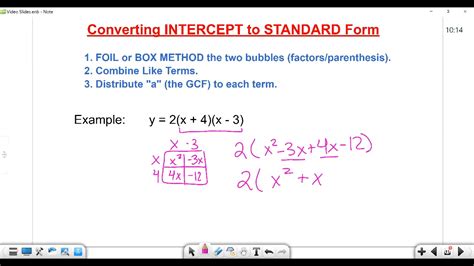
Converting Intercept Form to Standard Form: A Step-by-Step Guide

Now that we have a solid understanding of both forms, let's explore the conversion process.

Step 1: Identify the Slope (m) and Y-Intercept (b)

Take the intercept form equation and identify the values of m and b.

Step 2: Multiply Both Sides by -1

Multiply both sides of the equation by -1 to eliminate the negative sign in front of the x term.

Step 3: Rearrange the Equation

Rearrange the equation to isolate the x term on one side and the constant term on the other side.

Step 4: Write the Equation in Standard Form

Write the equation in standard form by combining the x and y terms on the left-hand side and the constant term on the right-hand side.

Example: Converting Intercept Form to Standard Form

Suppose we have the intercept form equation:

y = 2x + 3

Step 1: Identify the Slope (m) and Y-Intercept (b)

m = 2, b = 3

Step 2: Multiply Both Sides by -1

-2x - 3 = -y

Step 3: Rearrange the Equation

-2x + y = -3

Step 4: Write the Equation in Standard Form

2x - y = 3

Common Challenges and Solutions

When converting intercept form to standard form, you may encounter some common challenges. Here are some solutions to help you overcome these obstacles:

• Slope-Intercept Form with a Negative Slope: When the slope is negative, you may need to adjust the signs of the x and y terms accordingly.
• Y-Intercept with a Fraction: When the y-intercept is a fraction, you may need to multiply both sides of the equation by the denominator to eliminate the fraction.

Conclusion

Converting intercept form to standard form is a fundamental skill in mathematics, and with practice, you can master it. Remember to identify the slope and y-intercept, multiply both sides by -1, rearrange the equation, and write the equation in standard form. By following these steps and overcoming common challenges, you'll become proficient in converting linear equations with ease.

Get Involved and Share Your Thoughts

Now that you've learned how to convert intercept form to standard form, we'd love to hear from you! Share your thoughts, ask questions, or provide feedback in the comments section below. Let's create a community that fosters learning and growth.