Convert Equations To Slope Intercept Form Easily

Quick Links :

Converting equations to slope-intercept form is a crucial skill in mathematics, particularly in algebra and geometry. Slope-intercept form, denoted as y = mx + b, is a way of expressing linear equations where m represents the slope of the line and b represents the y-intercept. This form makes it easier to understand the behavior of the line, including its steepness and the point at which it intersects the y-axis.

Mastering the conversion of equations to slope-intercept form can significantly enhance problem-solving skills in various mathematical contexts. Whether you're dealing with word problems, graphing lines, or solving systems of equations, understanding how to convert equations to slope-intercept form is a valuable tool.

Importance of Slope-Intercept Form

The slope-intercept form, y = mx + b, offers a straightforward way to analyze lines. The slope, m, indicates how steep the line is and whether it rises or falls as you move from left to right. A positive slope indicates a line that rises, while a negative slope indicates a line that falls. The y-intercept, b, is the point where the line crosses the y-axis, providing valuable information about the line's position relative to the coordinate plane.

This form is particularly useful in real-world applications where linear relationships need to be understood or predicted. For example, in economics, the slope-intercept form can be used to model supply and demand curves, while in physics, it can be used to describe the motion of objects under constant acceleration.

Steps to Convert Equations to Slope-Intercept Form

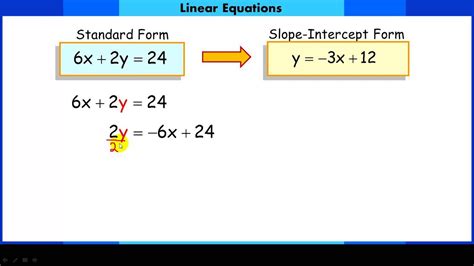
Converting equations to slope-intercept form involves a series of steps that help in rearranging the equation to isolate y on one side of the equation.

1. Start with the given equation: Begin with the equation you want to convert. It might be in standard form (Ax + By = C), point-slope form (y - y1 = m(x - x1)), or another form.

2. Rearrange the equation to solve for y: Move all terms involving x to one side and constants to the other side. This might involve adding or subtracting the same value to both sides to maintain the equation's balance.

3. Isolate y: Once you've rearranged the equation, ensure y is isolated on one side. This might require factoring or dividing by a coefficient if y is multiplied by a number other than 1.

4. Write the equation in slope-intercept form: After isolating y, the equation should be in slope-intercept form, y = mx + b. Identify the slope (m) and the y-intercept (b) from the equation.

Example: Converting an Equation to Slope-Intercept Form

Given the equation 2x + 3y = 6, convert it to slope-intercept form.

• Rearrange the equation: Subtract 2x from both sides to get the terms involving x on one side: 3y = -2x + 6.

• Isolate y: Divide both sides by 3 to isolate y: y = (-2/3)x + 2.

• Identify the slope and y-intercept: The slope (m) is -2/3, and the y-intercept (b) is 2.

Tips for Converting Equations

• Pay attention to signs: When rearranging the equation, ensure you correctly handle the signs of the terms. A mistake in the sign can lead to an incorrect slope or y-intercept.

• Simplify the equation: Once in slope-intercept form, simplify the equation if possible. This includes reducing fractions or combining like terms.

• Check your work: Plug the original values back into the converted equation to ensure it holds true.

Conclusion: Enhancing Your Math Skills

Converting equations to slope-intercept form is a fundamental skill that opens doors to deeper understanding and manipulation of linear equations. By mastering this conversion, you enhance your ability to solve a wide range of mathematical problems, from simple algebra to complex graphing and analysis. Practice regularly and apply these skills to various problems to solidify your understanding of slope-intercept form.