General Form Of The Equation Explained Simply

Quick Links :

The general form of an equation is a fundamental concept in mathematics, and it's essential to understand it to solve various mathematical problems. In this article, we'll break down the general form of an equation in simple terms, making it easy for you to grasp.

What is an Equation?

An equation is a statement that expresses the equality of two mathematical expressions, often containing variables and constants. It's a way to describe a relationship between different values. Equations can be simple or complex, but they all follow a specific structure.

General Form of an Equation

The general form of an equation is a way to represent any equation in a standardized format. It's written as:

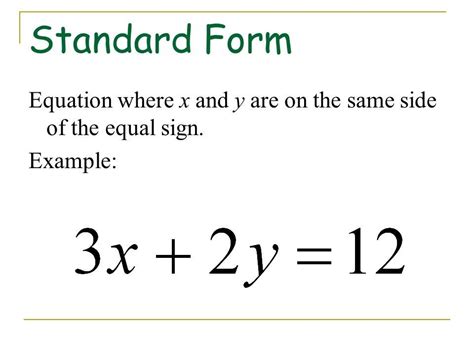
ax + by = c

Where:

• a and b are coefficients (numbers) that are multiplied by the variables
• x and y are variables (unknown values)
• c is a constant (a number)

This format is useful because it allows us to identify the coefficients, variables, and constants in an equation, making it easier to solve.

Understanding the Components

Let's break down the components of the general form:

• Coefficients (a and b): These are numbers that are multiplied by the variables. They can be positive, negative, or zero.
• Variables (x and y): These are unknown values that we're trying to solve for. They can be letters, symbols, or even words.
• Constant (c): This is a number that's not multiplied by a variable. It's a fixed value that we know.

Types of Equations

There are several types of equations, including:

• Linear equations: These are equations in which the highest power of the variable is 1. For example, 2x + 3 = 5.
• Quadratic equations: These are equations in which the highest power of the variable is 2. For example, x^2 + 4x + 4 = 0.
• Polynomial equations: These are equations in which the highest power of the variable is 3 or more. For example, x^3 + 2x^2 - 7x - 12 = 0.

Solving Equations

Solving an equation means finding the value of the variable that makes the equation true. There are several methods to solve equations, including:

• Addition and subtraction: Adding or subtracting the same value to both sides of the equation.
• Multiplication and division: Multiplying or dividing both sides of the equation by the same value.
• Factoring: Breaking down the equation into simpler factors.

Example Problem

Let's solve a simple equation using the general form:

2x + 5 = 11

To solve for x, we need to isolate the variable. We can do this by subtracting 5 from both sides:

2x = 11 - 5 2x = 6

Next, we can divide both sides by 2 to solve for x:

x = 6/2 x = 3

Real-World Applications

Equations have numerous real-world applications, including:

• Physics: Equations are used to describe the laws of motion, energy, and gravity.
• Engineering: Equations are used to design and optimize systems, such as bridges and electronic circuits.
• Economics: Equations are used to model economic systems and make predictions about market trends.

Conclusion

In conclusion, the general form of an equation is a powerful tool for solving mathematical problems. By understanding the components of an equation and how to manipulate them, we can solve a wide range of equations and apply them to real-world problems.

We hope this article has helped you understand the general form of an equation and how to solve it. Do you have any questions or topics you'd like to discuss? Share your thoughts in the comments below!