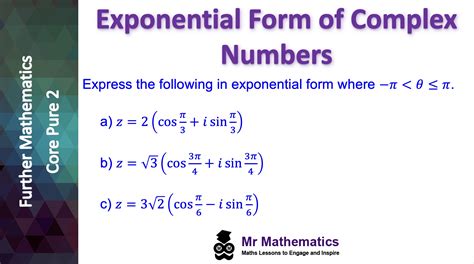
Exponential form of square root is a powerful tool for simplifying complex numbers. With the rise of advanced mathematical concepts and computational tools, understanding the exponential form of square root has become more crucial than ever. In this article, we will delve into the world of exponential form of square root, exploring its definition, benefits, and practical applications.
Mathematicians and scientists have long been fascinated by the concept of square roots. From ancient civilizations to modern-day researchers, the pursuit of understanding and simplifying complex numbers has led to numerous breakthroughs. The exponential form of square root is a significant milestone in this journey, offering a more intuitive and efficient way to work with complex numbers.
What is Exponential Form of Square Root?

The exponential form of square root is a mathematical representation that expresses a square root in terms of an exponential function. This form is particularly useful when dealing with complex numbers, as it provides a more straightforward and elegant way to simplify and manipulate these numbers.
In essence, the exponential form of square root involves expressing a square root as a power of a complex number. This power is typically represented as a fraction, where the numerator is the exponent and the denominator is the base. By converting a square root to its exponential form, mathematicians and scientists can take advantage of the properties of exponents to simplify complex calculations.
Benefits of Exponential Form of Square Root

The exponential form of square root offers several benefits that make it an indispensable tool in mathematics and science. Some of the key advantages include:
- Simplified calculations: By expressing a square root in its exponential form, complex calculations can be significantly simplified. This is particularly useful when working with complex numbers, where calculations can quickly become cumbersome.
- Improved accuracy: The exponential form of square root provides a more precise representation of complex numbers, reducing the risk of errors and inaccuracies.
- Enhanced intuition: The exponential form of square root offers a more intuitive understanding of complex numbers, allowing mathematicians and scientists to better comprehend the underlying relationships and patterns.
- Increased flexibility: The exponential form of square root provides a flexible framework for working with complex numbers, enabling the use of various mathematical techniques and tools.
Working with Exponential Form of Square Root

To work with the exponential form of square root, mathematicians and scientists need to understand the underlying principles and techniques. Here are some key steps to get started:
- Express the square root in its exponential form: This involves rewriting the square root as a power of a complex number.
- Simplify the expression: Use the properties of exponents to simplify the expression and reduce the complexity of the calculation.
- Apply mathematical techniques: Utilize various mathematical techniques, such as algebraic manipulation and calculus, to further simplify and analyze the expression.
Example: Simplifying a Complex Number using Exponential Form of Square Root
Consider the complex number √(3+4i). To simplify this number using the exponential form of square root, we can express it as:
√(3+4i) = (3+4i)^(1/2)
Using the properties of exponents, we can simplify this expression as:
(3+4i)^(1/2) = 2^(1/2) * (1+i)^(1/2)
This expression can be further simplified using various mathematical techniques, such as algebraic manipulation and calculus.
Practical Applications of Exponential Form of Square Root

The exponential form of square root has numerous practical applications in mathematics, science, and engineering. Some of the key areas where this concept is used include:
- Signal processing: The exponential form of square root is used in signal processing to simplify complex calculations and improve the accuracy of results.
- Control systems: The exponential form of square root is used in control systems to analyze and design complex systems.
- Quantum mechanics: The exponential form of square root is used in quantum mechanics to simplify complex calculations and provide a more intuitive understanding of quantum systems.
Conclusion: Unlocking the Power of Exponential Form of Square Root
The exponential form of square root is a powerful tool for simplifying complex numbers and unlocking the secrets of mathematics and science. By understanding the definition, benefits, and practical applications of this concept, mathematicians and scientists can gain a deeper insight into the underlying principles and patterns that govern our world.
As we continue to explore the vast expanse of mathematical knowledge, the exponential form of square root remains an essential tool in our arsenal. Whether you are a student, researcher, or professional, mastering this concept can help you unlock new insights and achieve greater success in your endeavors.
What is the exponential form of square root?
+The exponential form of square root is a mathematical representation that expresses a square root in terms of an exponential function.
What are the benefits of using the exponential form of square root?
+The exponential form of square root offers several benefits, including simplified calculations, improved accuracy, enhanced intuition, and increased flexibility.
How is the exponential form of square root used in practical applications?
+The exponential form of square root is used in various practical applications, including signal processing, control systems, and quantum mechanics.