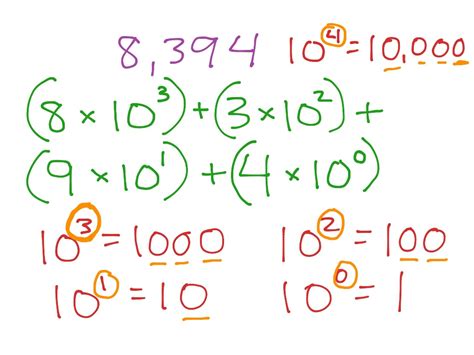5 Ways To Write Exponents In Expanded Form

Quick Links :

Exponents are a fundamental concept in mathematics, and being able to write them in expanded form is an essential skill for students to master. Writing exponents in expanded form involves expressing the repeated multiplication of a number in a more compact and readable way. In this article, we will explore five ways to write exponents in expanded form, along with examples and practical exercises to help you reinforce your understanding.

What are Exponents?

Exponents are shorthand notations used to represent repeated multiplication of a number. For example, 2^3 is equal to 2 multiplied by itself three times: 2 × 2 × 2. Exponents can be positive or negative, and they can be used to represent a wide range of mathematical operations.

Method 1: Using Repeated Multiplication

One way to write exponents in expanded form is to use repeated multiplication. This involves multiplying the base number by itself as many times as indicated by the exponent.

Example: Write 2^4 in expanded form.

Solution: 2^4 = 2 × 2 × 2 × 2 = 16

Practical Exercise:

Write the following exponents in expanded form using repeated multiplication:

• 3^2
• 4^3
• 5^2

Method 2: Using the Laws of Exponents

Another way to write exponents in expanded form is to use the laws of exponents. The laws of exponents state that when you multiply two numbers with the same base, you add the exponents.

Example: Write 2^3 × 2^2 in expanded form.

Solution: 2^3 × 2^2 = 2^(3+2) = 2^5 = 2 × 2 × 2 × 2 × 2 = 32

Practical Exercise:

Write the following exponents in expanded form using the laws of exponents:

• 3^2 × 3^3
• 4^2 × 4^4
• 5^3 × 5^2

Method 3: Using the Product Rule

The product rule is another method for writing exponents in expanded form. This rule states that when you multiply two numbers with different bases, you multiply the numbers and add the exponents.

Example: Write 2^3 × 3^2 in expanded form.

Solution: 2^3 × 3^2 = (2 × 2 × 2) × (3 × 3) = 72

Practical Exercise:

Write the following exponents in expanded form using the product rule:

• 2^2 × 3^3
• 4^3 × 5^2
• 3^2 × 2^4

Method 4: Using the Power Rule

The power rule is a method for writing exponents in expanded form that involves raising a power to another power.

Example: Write (2^3)^2 in expanded form.

Solution: (2^3)^2 = 2^(3×2) = 2^6 = 2 × 2 × 2 × 2 × 2 × 2 = 64

Practical Exercise:

Write the following exponents in expanded form using the power rule:

• (3^2)^3
• (4^3)^2
• (5^2)^4

Method 5: Using the Quotient Rule

The quotient rule is a method for writing exponents in expanded form that involves dividing two numbers with the same base.

Example: Write 2^4 ÷ 2^2 in expanded form.

Solution: 2^4 ÷ 2^2 = 2^(4-2) = 2^2 = 2 × 2 = 4

Practical Exercise:

Write the following exponents in expanded form using the quotient rule:

• 3^3 ÷ 3^2
• 4^4 ÷ 4^3
• 5^5 ÷ 5^3

Now that you have learned five ways to write exponents in expanded form, it's time to put your skills to the test! Try practicing with different types of exponents and see how well you can apply these methods. Remember to take your time and work carefully, as writing exponents in expanded form can be a challenging task.