Complex Numbers Exponential Form Explained Simply

Quick Links :

In the realm of mathematics, complex numbers are a fundamental concept that has far-reaching implications in various fields, including algebra, geometry, and engineering. One of the most powerful and fascinating aspects of complex numbers is their exponential form, which provides a unique and insightful way of representing and working with these numbers. In this article, we will delve into the world of complex numbers exponential form, exploring its definition, significance, and applications, as well as providing practical examples and explanations to help readers grasp this concept with ease.

What are Complex Numbers?

Before we dive into the exponential form of complex numbers, it's essential to understand what complex numbers are. In simple terms, complex numbers are numbers that can be expressed in the form a + bi, where a and b are real numbers, and i is the imaginary unit, which satisfies the equation i^2 = -1. The real part of the complex number is a, and the imaginary part is bi.

Exponential Form of Complex Numbers

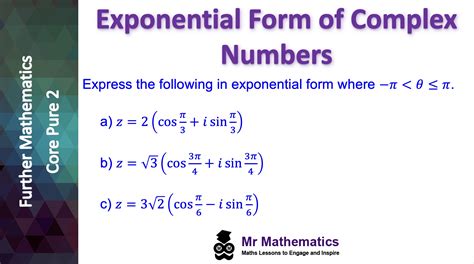
The exponential form of complex numbers is a representation of complex numbers using the exponential function, which is defined as e^(iθ) = cos(θ) + i sin(θ), where θ is the angle in radians. This representation is also known as Euler's formula, named after the Swiss mathematician Leonhard Euler. Using this formula, we can express any complex number in the form re^(iθ), where r is the magnitude (or length) of the complex number, and θ is its argument (or angle).

Why is the Exponential Form Important?

The exponential form of complex numbers has far-reaching implications in various fields, including mathematics, physics, and engineering. Some of the key benefits of this representation include:

• Simplifies calculations: The exponential form makes it easier to perform calculations with complex numbers, especially when dealing with powers and roots.
• Provides geometric insight: The exponential form helps to visualize complex numbers in the complex plane, which is essential for understanding many mathematical and physical concepts.
• Facilitates mathematical derivations: The exponential form is used to derive many mathematical formulas and theorems, including the fundamental theorem of algebra and the Cauchy-Riemann equations.

How to Convert Complex Numbers to Exponential Form

Converting complex numbers to exponential form involves finding the magnitude and argument of the complex number. Here are the steps:

1. Find the magnitude (r) of the complex number using the formula r = √(a^2 + b^2), where a and b are the real and imaginary parts of the complex number.
2. Find the argument (θ) of the complex number using the formula θ = arctan(b/a), where a and b are the real and imaginary parts of the complex number.
3. Express the complex number in exponential form as re^(iθ).

Examples of Converting Complex Numbers to Exponential Form

• Example 1: Convert the complex number 3 + 4i to exponential form. Solution: Find the magnitude (r) and argument (θ) of the complex number: r = √(3^2 + 4^2) = 5 θ = arctan(4/3) = 0.927 radians Express the complex number in exponential form: 5e^(i0.927)
• Example 2: Convert the complex number -2 - 5i to exponential form. Solution: Find the magnitude (r) and argument (θ) of the complex number: r = √((-2)^2 + (-5)^2) = √(29) θ = arctan(-5/-2) = -1.107 radians Express the complex number in exponential form: √(29)e^(-i1.107)

Applications of Complex Numbers Exponential Form

The exponential form of complex numbers has numerous applications in various fields, including:

• Electrical engineering: Complex numbers are used to analyze and design electrical circuits, including impedance and resonance.
• Signal processing: Complex numbers are used to represent and analyze signals in fields such as audio processing and image processing.
• Quantum mechanics: Complex numbers are used to represent wave functions and probability amplitudes in quantum mechanics.

Real-World Examples of Complex Numbers Exponential Form

• Example 1: Analyzing an electrical circuit using complex numbers. In an electrical circuit, the impedance (Z) is given by the formula Z = R + iX, where R is the resistance and X is the reactance. Using the exponential form, we can express the impedance as Ze^(iθ), where Z is the magnitude and θ is the argument.
• Example 2: Representing a signal using complex numbers. In signal processing, a signal can be represented using complex numbers, where the real part represents the amplitude and the imaginary part represents the phase. Using the exponential form, we can express the signal as Ae^(iωt), where A is the amplitude, ω is the frequency, and t is time.

Conclusion

In conclusion, the exponential form of complex numbers is a powerful and fascinating representation that provides a unique and insightful way of working with complex numbers. By understanding the exponential form, we can simplify calculations, gain geometric insight, and facilitate mathematical derivations. The applications of complex numbers exponential form are numerous, and it has far-reaching implications in various fields, including electrical engineering, signal processing, and quantum mechanics.

We hope this article has provided a comprehensive and accessible explanation of complex numbers exponential form. If you have any questions or comments, please feel free to share them below.