Simplify Fractions In 5 Easy Steps

Quick Links :

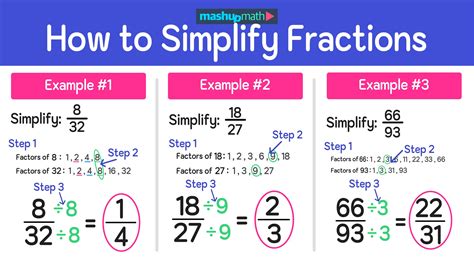
Simplifying fractions is a fundamental concept in mathematics that can seem daunting at first, but with the right approach, it can be made easy and accessible to everyone. Whether you're a student struggling with fractions or an adult looking to brush up on your math skills, this article will guide you through the process of simplifying fractions in 5 easy steps.

In everyday life, fractions are used to represent parts of a whole, such as 1/2, 1/4, or 3/4. However, when working with fractions, it's essential to simplify them to their lowest terms to make calculations easier and more efficient. In this article, we'll explore the 5 easy steps to simplify fractions and provide examples to help you understand the concept better.

Step 1: Identify the Greatest Common Divisor (GCD)

The first step in simplifying a fraction is to identify the greatest common divisor (GCD) of the numerator and denominator. The GCD is the largest number that divides both the numerator and denominator without leaving a remainder. To find the GCD, you can use the prime factorization method or the Euclidean algorithm.

For example, let's consider the fraction 12/18. To simplify this fraction, we need to find the GCD of 12 and 18.

Prime Factorization Method

Using the prime factorization method, we can break down 12 and 18 into their prime factors:

12 = 2 × 2 × 3 18 = 2 × 3 × 3

As we can see, both 12 and 18 share a common factor of 2 and 3. Therefore, the GCD of 12 and 18 is 6.

Step 2: Divide the Numerator and Denominator by the GCD

Once you've identified the GCD, the next step is to divide both the numerator and denominator by the GCD. This will simplify the fraction to its lowest terms.

Using the example above, we can simplify the fraction 12/18 by dividing both the numerator and denominator by 6:

12 ÷ 6 = 2 18 ÷ 6 = 3

The simplified fraction is 2/3.

Step 3: Check for Any Remaining Common Factors

After simplifying the fraction, it's essential to check if there are any remaining common factors between the numerator and denominator. If there are, you'll need to repeat the process of finding the GCD and dividing both the numerator and denominator.

For example, let's consider the fraction 8/12. After simplifying, we get 2/3. However, we can see that both 2 and 3 share a common factor of 1. Since 1 is the smallest unit, we can't simplify the fraction further.

Step 4: Write the Simplified Fraction

Once you've checked for any remaining common factors, you can write the simplified fraction.

Using the example above, the simplified fraction is 2/3.

Step 5: Verify the Simplified Fraction

The final step is to verify the simplified fraction by checking if it's equivalent to the original fraction.

Using the example above, we can verify the simplified fraction 2/3 by multiplying both the numerator and denominator by 6:

2 × 6 = 12 3 × 6 = 18

As we can see, the simplified fraction 2/3 is equivalent to the original fraction 12/18.

In conclusion, simplifying fractions is a straightforward process that involves identifying the greatest common divisor (GCD) of the numerator and denominator, dividing both the numerator and denominator by the GCD, checking for any remaining common factors, writing the simplified fraction, and verifying the simplified fraction.