Fractions are a fundamental concept in mathematics, and simplifying them is an essential skill for anyone working with numbers. Whether you're a student, a teacher, or just someone who wants to improve your math skills, learning to simplify fractions is a crucial step in building a strong foundation in mathematics. In this article, we'll explore the importance of simplifying fractions, and provide a simple 2-step process to simplify fractions with ease.
The Importance of Simplifying Fractions
Simplifying fractions is a crucial step in many mathematical operations, such as addition, subtraction, multiplication, and division. When fractions are not simplified, they can be difficult to work with, and can lead to errors and confusion. Simplifying fractions helps to:
- Reduce errors: Simplified fractions are easier to work with, reducing the likelihood of errors and mistakes.
- Improve understanding: Simplified fractions make it easier to understand mathematical concepts and relationships.
- Enhance problem-solving: Simplified fractions enable you to solve problems more efficiently and effectively.

2 Easy Steps to Simplify Fractions
Simplifying fractions is a straightforward process that involves two easy steps:
Step 1: Find the Greatest Common Divisor (GCD)
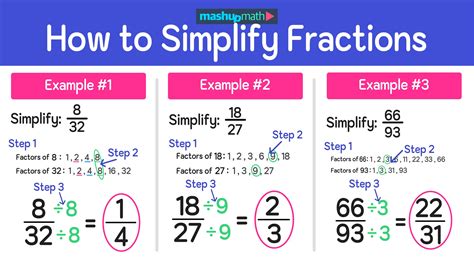
The first step in simplifying a fraction is to find the greatest common divisor (GCD) of the numerator and denominator. The GCD is the largest number that divides both numbers without leaving a remainder.
For example, let's say we want to simplify the fraction 12/18. To find the GCD, we can list the factors of both numbers:
- Factors of 12: 1, 2, 3, 4, 6, 12
- Factors of 18: 1, 2, 3, 6, 9, 18
The largest number that appears in both lists is 6, which is the GCD of 12 and 18.
Step 2: Divide Both Numbers by the GCD
Once we have found the GCD, we can simplify the fraction by dividing both the numerator and denominator by the GCD.
Using the example above, we can simplify the fraction 12/18 by dividing both numbers by 6:
- Numerator: 12 ÷ 6 = 2
- Denominator: 18 ÷ 6 = 3
The simplified fraction is 2/3.
Types of Fractions
There are several types of fractions, including:
Proper Fractions
A proper fraction is a fraction where the numerator is less than the denominator.
Example: 3/4
Improper Fractions
An improper fraction is a fraction where the numerator is greater than or equal to the denominator.
Example: 5/3
Mixed Numbers
A mixed number is a combination of a whole number and a proper fraction.
Example: 2 3/4
Simplifying Proper Fractions
Simplifying proper fractions is a straightforward process that involves finding the GCD and dividing both numbers by the GCD.
Example: Simplify the fraction 8/12
- Find the GCD: 4
- Divide both numbers by the GCD: 8 ÷ 4 = 2, 12 ÷ 4 = 3
- Simplified fraction: 2/3
Simplifying Improper Fractions
Simplifying improper fractions involves finding the GCD and dividing both numbers by the GCD. However, since the numerator is greater than or equal to the denominator, we may need to convert the improper fraction to a mixed number.
Example: Simplify the fraction 9/4
- Find the GCD: 1
- Divide both numbers by the GCD: 9 ÷ 1 = 9, 4 ÷ 1 = 4
- Convert to a mixed number: 2 1/4
Simplifying Mixed Numbers
Simplifying mixed numbers involves converting the mixed number to an improper fraction, finding the GCD, and dividing both numbers by the GCD.
Example: Simplify the mixed number 3 2/5
- Convert to an improper fraction: 17/5
- Find the GCD: 1
- Divide both numbers by the GCD: 17 ÷ 1 = 17, 5 ÷ 1 = 5
- Simplified fraction: 17/5

Common Mistakes to Avoid
When simplifying fractions, there are several common mistakes to avoid:
- Forgetting to find the GCD
- Dividing both numbers by the wrong number
- Not converting improper fractions to mixed numbers
- Not converting mixed numbers to improper fractions
Real-World Applications
Simplifying fractions has numerous real-world applications, including:
Cooking and Recipes
Simplifying fractions is essential in cooking and recipes, where measurements need to be accurate and precise.
Example: A recipe calls for 3/4 cup of flour, but you only have a 1/2 cup measuring cup. Simplifying the fraction 3/4 to 2/3 enables you to measure the correct amount of flour.
Science and Engineering
Simplifying fractions is crucial in science and engineering, where precise calculations are necessary.
Example: A scientist needs to calculate the ratio of a substance's weight to its volume. Simplifying the fraction enables the scientist to make accurate calculations.
Finance and Accounting
Simplifying fractions is essential in finance and accounting, where financial calculations need to be accurate and precise.
Example: A financial analyst needs to calculate the ratio of a company's profits to its expenses. Simplifying the fraction enables the analyst to make accurate calculations.

Conclusion
Simplifying fractions is a fundamental skill that is essential in many mathematical operations. By following the two easy steps outlined in this article, you can simplify fractions with ease and accuracy. Whether you're a student, teacher, or professional, simplifying fractions is a crucial step in building a strong foundation in mathematics.
We hope you found this article informative and helpful. Share your thoughts and comments below!
What is the greatest common divisor (GCD)?
+The greatest common divisor (GCD) is the largest number that divides both numbers without leaving a remainder.
How do I simplify a mixed number?
+To simplify a mixed number, convert it to an improper fraction, find the GCD, and divide both numbers by the GCD.
What is the difference between a proper fraction and an improper fraction?
+A proper fraction is a fraction where the numerator is less than the denominator, while an improper fraction is a fraction where the numerator is greater than or equal to the denominator.