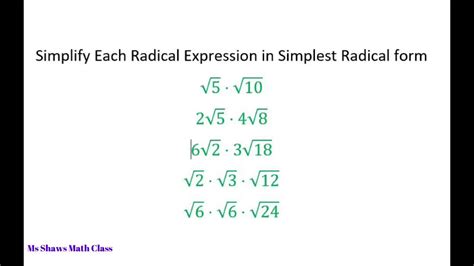
Simplifying Radicals To Lowest Terms: 128 In Simplest Form

Quick Links :

Simplifying radicals to their lowest terms is an essential concept in mathematics, particularly in algebra and geometry. It involves expressing a radical expression in its most basic form, which can be helpful in various mathematical operations. In this article, we will explore the concept of simplifying radicals, with a focus on the radical 128 in its simplest form.

What are Radicals?

Radicals, also known as roots, are mathematical expressions that represent a number raised to a fractional power. For example, √x represents the square root of x, while ³√x represents the cube root of x. Radicals are used to solve equations and to simplify complex mathematical expressions.

Why Simplify Radicals?

Simplifying radicals is important for several reasons:

• It makes mathematical expressions more manageable and easier to work with.
• It helps to avoid errors in mathematical calculations.
• It allows for the comparison of different radical expressions.

Simplifying Radicals: A Step-by-Step Guide

To simplify a radical expression, follow these steps:

1. Find the largest perfect square or perfect cube that divides the radicand: The radicand is the number under the radical sign. Look for the largest perfect square or perfect cube that divides the radicand evenly.
2. Write the radicand as a product of the perfect square or perfect cube and another factor: Express the radicand as a product of the perfect square or perfect cube and another factor.
3. Take the square root or cube root of the perfect square or perfect cube: Take the square root or cube root of the perfect square or perfect cube.
4. Leave the remaining factor under the radical sign: Leave the remaining factor under the radical sign.

Example: Simplifying the Radical 128

Let's simplify the radical 128:

1. Find the largest perfect square or perfect cube that divides 128: The largest perfect square that divides 128 is 64, which is 8².
2. Write 128 as a product of 64 and another factor: Express 128 as 64 × 2.
3. Take the square root of 64: Take the square root of 64, which is 8.
4. Leave the remaining factor under the radical sign: Leave the remaining factor, 2, under the radical sign.

Therefore, √128 = 8√2.

Key Concepts and Formulas

Here are some key concepts and formulas related to simplifying radicals:

• Perfect squares: A perfect square is a number that can be expressed as the product of an integer and itself. For example, 16 is a perfect square because it can be expressed as 4 × 4.
• Perfect cubes: A perfect cube is a number that can be expressed as the product of an integer and itself twice. For example, 27 is a perfect cube because it can be expressed as 3 × 3 × 3.
• Square root: The square root of a number is a value that, when multiplied by itself, gives the original number. For example, the square root of 16 is 4 because 4 × 4 = 16.
• Cube root: The cube root of a number is a value that, when multiplied by itself twice, gives the original number. For example, the cube root of 27 is 3 because 3 × 3 × 3 = 27.

Tips and Tricks

Here are some tips and tricks for simplifying radicals:

• Look for perfect squares and perfect cubes: Always look for perfect squares and perfect cubes when simplifying radicals.
• Use the multiplication property of radicals: The multiplication property of radicals states that √ab = √a × √b.
• Use the division property of radicals: The division property of radicals states that √a/√b = √(a/b).

Applications of Simplifying Radicals

Simplifying radicals has several applications in mathematics and real-life situations:

• Algebra: Simplifying radicals is essential in algebra, particularly when solving equations and manipulating expressions.
• Geometry: Simplifying radicals is used in geometry to calculate distances, areas, and volumes.
• Physics: Simplifying radicals is used in physics to calculate quantities such as velocity, acceleration, and force.

Conclusion

In conclusion, simplifying radicals is an important concept in mathematics that involves expressing a radical expression in its most basic form. By following the steps outlined in this article, you can simplify radicals with ease. Remember to look for perfect squares and perfect cubes, use the multiplication and division properties of radicals, and apply simplifying radicals to real-life situations.