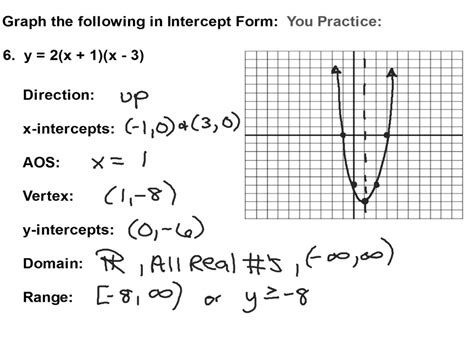
Unlocking the Secrets of X-Intercept Form Quadratics

Quadratic equations are a fundamental concept in algebra, and understanding their various forms is essential for solving problems in mathematics and real-world applications. One of the most powerful forms of quadratic equations is the x-intercept form, also known as the factored form. In this article, we will explore the x-intercept form of quadratics and provide a step-by-step guide on how to master it in 5 easy steps.
What is X-Intercept Form?
The x-intercept form of a quadratic equation is a way of expressing the equation in terms of its x-intercepts, which are the points where the graph of the quadratic function crosses the x-axis. This form is particularly useful for solving quadratic equations, graphing quadratic functions, and analyzing the behavior of quadratic systems.
Step 1: Understanding the General Form of Quadratics

Before diving into the x-intercept form, it's essential to understand the general form of a quadratic equation, which is:
ax^2 + bx + c = 0
where a, b, and c are constants, and x is the variable. This form is also known as the standard form of a quadratic equation.
Why is the General Form Important?
The general form is crucial because it provides a framework for expressing quadratic equations in a consistent and standardized way. It's also the basis for transforming quadratic equations into other forms, such as the x-intercept form.
Step 2: Finding the X-Intercepts

To convert a quadratic equation into x-intercept form, we need to find the x-intercepts of the graph. The x-intercepts are the points where the graph crosses the x-axis, and they can be found by setting the equation equal to zero and solving for x.
For example, consider the quadratic equation:
x^2 + 5x + 6 = 0
To find the x-intercepts, we can factor the equation as:
(x + 2)(x + 3) = 0
This tells us that the x-intercepts are x = -2 and x = -3.
How to Find X-Intercepts
There are several ways to find x-intercepts, including:
- Factoring the quadratic equation
- Using the quadratic formula
- Graphing the quadratic function
Step 3: Expressing Quadratics in X-Intercept Form

Once we have found the x-intercepts, we can express the quadratic equation in x-intercept form. The x-intercept form of a quadratic equation is:
a(x - p)(x - q) = 0
where p and q are the x-intercepts, and a is a constant.
For example, the quadratic equation:
x^2 + 5x + 6 = 0
can be expressed in x-intercept form as:
(x + 2)(x + 3) = 0
Benefits of X-Intercept Form
The x-intercept form has several benefits, including:
- Easy to factor and solve
- Provides a clear visualization of the graph
- Useful for analyzing the behavior of quadratic systems
Step 4: Solving Quadratics in X-Intercept Form

Solving quadratics in x-intercept form is relatively straightforward. We can set each factor equal to zero and solve for x.
For example, consider the quadratic equation:
(x + 2)(x + 3) = 0
We can set each factor equal to zero and solve for x:
x + 2 = 0 --> x = -2 x + 3 = 0 --> x = -3
Common Mistakes to Avoid
When solving quadratics in x-intercept form, be careful to avoid common mistakes, such as:
- Forgetting to set each factor equal to zero
- Not solving for x correctly
Step 5: Graphing Quadratics in X-Intercept Form

Graphing quadratics in x-intercept form is also relatively straightforward. We can use the x-intercepts to sketch the graph.
For example, consider the quadratic equation:
(x + 2)(x + 3) = 0
We can use the x-intercepts to sketch the graph:
- Plot the points (-2, 0) and (-3, 0) on the x-axis
- Draw a smooth curve through the points to create the graph
Common Graphing Mistakes
When graphing quadratics in x-intercept form, be careful to avoid common mistakes, such as:
- Not plotting the x-intercepts correctly
- Not drawing a smooth curve through the points
What is the main advantage of using x-intercept form?
+The main advantage of using x-intercept form is that it provides a clear visualization of the graph and makes it easy to factor and solve.
How do I find the x-intercepts of a quadratic equation?
+You can find the x-intercepts of a quadratic equation by setting the equation equal to zero and solving for x. You can also use the quadratic formula or graph the quadratic function.
What is the difference between x-intercept form and standard form?
+X-intercept form is a way of expressing a quadratic equation in terms of its x-intercepts, while standard form is the general form of a quadratic equation.
By following these 5 easy steps, you can master the x-intercept form of quadratics and become proficient in solving and graphing quadratic equations. Remember to practice regularly and avoid common mistakes to become a pro in algebra. Share your thoughts and experiences in the comments below, and don't forget to share this article with your friends and classmates.