Unlocking the Power of Complex Numbers: An Introduction to Trigonometric Form

In the realm of mathematics, complex numbers are a fundamental concept that can be daunting for many students. However, with the right approach, understanding complex numbers can become a breeze. One of the most powerful ways to represent complex numbers is through the trigonometric form, which offers a unique perspective on these mathematical entities. In this article, we will delve into the world of trigonometric form of complex numbers, exploring its benefits, working mechanisms, and practical applications.
The Importance of Complex Numbers

Before we dive into the trigonometric form, it's essential to understand the significance of complex numbers in mathematics and science. Complex numbers extend the real number system, allowing us to represent quantities that cannot be expressed on the traditional number line. They have numerous applications in various fields, including:
- Electrical engineering: Complex numbers are used to analyze AC circuits and represent impedance.
- Signal processing: Complex numbers are employed in filtering, modulation, and demodulation techniques.
- Quantum mechanics: Complex numbers are used to describe wave functions and probability amplitudes.
- Navigation: Complex numbers are used in GPS systems to calculate positions and velocities.
What is Trigonometric Form?

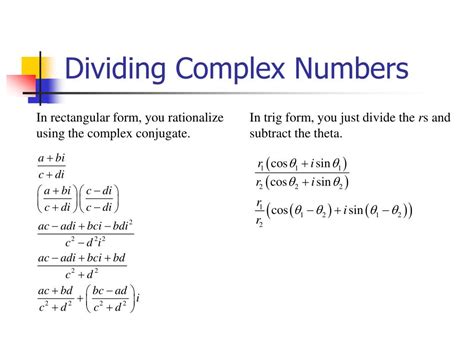
The trigonometric form of complex numbers represents a complex number as a sum of its magnitude (length) and angle (argument). This form is also known as the polar form. The trigonometric form is expressed as:
z = r(cosθ + isinθ)
where:
- z is the complex number
- r is the magnitude (length) of the complex number
- θ is the angle (argument) of the complex number
- cosθ is the cosine of the angle
- sinθ is the sine of the angle
- i is the imaginary unit (i = √(-1))
Benefits of Trigonometric Form
The trigonometric form offers several benefits, including:
- Simplified multiplication and division: When complex numbers are represented in trigonometric form, multiplication and division become much easier.
- Easier visualization: The trigonometric form allows us to visualize complex numbers as points on the complex plane, making it easier to understand their properties.
- Connection to trigonometry: The trigonometric form establishes a strong connection between complex numbers and trigonometry, enabling us to apply trigonometric concepts to complex number problems.
How to Convert Complex Numbers to Trigonometric Form

To convert a complex number to trigonometric form, follow these steps:
- Determine the magnitude (length) of the complex number using the formula:
r = √(a^2 + b^2)
where a and b are the real and imaginary parts of the complex number.
- Determine the angle (argument) of the complex number using the formula:
θ = arctan(b/a)
- Express the complex number in trigonometric form using the formula:
z = r(cosθ + isinθ)
Applications of Trigonometric Form

The trigonometric form has numerous applications in various fields, including:
- Electrical engineering: The trigonometric form is used to analyze AC circuits and represent impedance.
- Signal processing: The trigonometric form is employed in filtering, modulation, and demodulation techniques.
- Quantum mechanics: The trigonometric form is used to describe wave functions and probability amplitudes.
- Navigation: The trigonometric form is used in GPS systems to calculate positions and velocities.
Conclusion
In conclusion, the trigonometric form of complex numbers is a powerful tool that simplifies the representation and manipulation of complex numbers. By understanding the trigonometric form, you can unlock the secrets of complex numbers and apply them to various fields. We encourage you to explore the world of complex numbers and discover the many wonders that await you.
What is the main benefit of using the trigonometric form of complex numbers?
+The main benefit of using the trigonometric form is that it simplifies multiplication and division of complex numbers.
How do I convert a complex number to trigonometric form?
+To convert a complex number to trigonometric form, determine the magnitude (length) and angle (argument) using the formulas r = √(a^2 + b^2) and θ = arctan(b/a), and then express the complex number in trigonometric form using the formula z = r(cosθ + isinθ).
What are some applications of the trigonometric form of complex numbers?
+The trigonometric form has numerous applications in various fields, including electrical engineering, signal processing, quantum mechanics, and navigation.