Simplify The Ratio 15:25 To Fraction

Quick Links :

Simplifying Ratios to Fractions

Ratios and fractions are fundamental concepts in mathematics, often used to represent proportions and parts of a whole. Understanding how to simplify ratios to fractions is a crucial skill for various mathematical operations. In this article, we will explore how to simplify the ratio 15:25 to a fraction, providing a comprehensive guide on the process.

Understanding Ratios and Fractions

Before we dive into simplifying the ratio 15:25, it is essential to understand the basics of ratios and fractions. A ratio is a way of comparing two quantities by division, represented by a colon (:). For example, 15:25 represents the ratio of 15 to 25. A fraction, on the other hand, is a way of expressing a part of a whole as a numerical value, represented by a numerator (top number) and a denominator (bottom number), separated by a slash (/).

Why Simplify Ratios to Fractions?

Simplifying ratios to fractions is essential for various mathematical operations, such as addition, subtraction, multiplication, and division. Fractions provide a more straightforward way of representing proportions, making it easier to perform calculations and compare values. Additionally, simplifying ratios to fractions helps to reduce errors and improve accuracy in mathematical calculations.

The Simplification Process

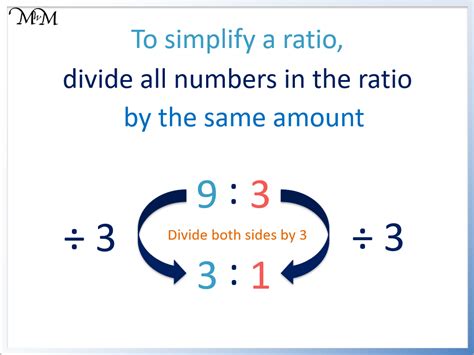
To simplify the ratio 15:25 to a fraction, we need to follow these steps:

1. Write the ratio as a fraction: To convert the ratio 15:25 to a fraction, we write it as 15/25.
2. Find the greatest common divisor (GCD): The GCD is the largest number that divides both 15 and 25 without leaving a remainder. In this case, the GCD is 5.
3. Divide both numbers by the GCD: To simplify the fraction, we divide both 15 and 25 by the GCD, which is 5. This gives us 15 ÷ 5 = 3 and 25 ÷ 5 = 5.
4. Write the simplified fraction: The simplified fraction is 3/5.

Example Calculation

Let's use the simplified fraction 3/5 to calculate a real-world example:

If a recipe requires 15 cups of flour and 25 cups of sugar, and we want to make half the recipe, how much flour and sugar do we need?

Using the simplified fraction 3/5, we can calculate the amount of flour and sugar needed:

Flour: 15 ÷ 2 = 7.5 cups Sugar: 25 ÷ 2 = 12.5 cups

However, since we simplified the ratio to 3/5, we can use this fraction to calculate the amount of flour and sugar needed:

Flour: 3/5 × 2.5 = 1.5 cups Sugar: 5/5 × 2.5 = 2.5 cups

As you can see, the simplified fraction 3/5 provides a more straightforward way of calculating the amount of flour and sugar needed for the recipe.

Practical Applications

Simplifying ratios to fractions has numerous practical applications in various fields, including:

• Cooking and recipes: Simplifying ratios to fractions helps in scaling recipes up or down, ensuring accurate proportions of ingredients.
• Finance and accounting: Fractions are used to represent percentages, interest rates, and investment returns, making it essential to simplify ratios to fractions for accurate calculations.
• Science and engineering: Fractions are used to represent proportions and quantities in scientific and engineering applications, such as chemistry, physics, and mathematics.

Conclusion

In conclusion, simplifying ratios to fractions is an essential skill in mathematics, providing a more straightforward way of representing proportions and performing calculations. By following the steps outlined in this article, you can simplify the ratio 15:25 to the fraction 3/5, and apply this skill to various practical applications. Remember to practice simplifying ratios to fractions regularly to improve your mathematical skills and accuracy.

What's your take on simplifying ratios to fractions? Share your thoughts and examples in the comments below!