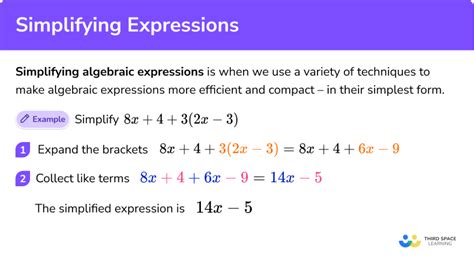
Simplifying expressions can be a daunting task, but with the right approach, it can be done in just one easy step. In this article, we will explore the concept of simplifying expressions, its importance, and provide a straightforward method to simplify expressions in no time.
Simplifying expressions is a fundamental concept in mathematics and algebra. It involves manipulating algebraic expressions to make them easier to understand and work with. Simplifying expressions can help to:
- Reduce errors by minimizing the complexity of the expression
- Improve understanding by breaking down complex expressions into simpler components
- Enhance problem-solving skills by providing a clear and concise representation of the expression
Despite its importance, many students and professionals struggle with simplifying expressions. The traditional method of simplifying expressions involves a series of steps, including expanding, factoring, and combining like terms. However, this approach can be time-consuming and prone to errors.
The One-Step Method to Simplify Expressions

The one-step method to simplify expressions involves using the concept of "grouping." Grouping is a technique that involves rearranging the terms of an expression to simplify it. This approach is based on the idea that expressions can be simplified by grouping like terms together.
To simplify an expression using the one-step method, follow these steps:
- Identify the like terms in the expression
- Group the like terms together
- Combine the like terms
For example, consider the expression: 2x + 3y + 4x - 2y
Using the one-step method, we can simplify this expression by grouping the like terms together:
(2x + 4x) + (3y - 2y)
= 6x + y
Benefits of the One-Step Method
The one-step method to simplify expressions offers several benefits, including:
- Reduced errors: By grouping like terms together, the one-step method minimizes the risk of errors.
- Improved understanding: The one-step method provides a clear and concise representation of the expression, making it easier to understand.
- Enhanced problem-solving skills: The one-step method provides a straightforward approach to simplifying expressions, enabling you to tackle complex problems with confidence.
Common Challenges and Solutions

Despite the benefits of the one-step method, some expressions can be challenging to simplify. Here are some common challenges and solutions:
-
Challenge: Simplifying expressions with multiple variables
-
Solution: Use the one-step method to group like terms together, and then simplify the resulting expression.
-
Challenge: Simplifying expressions with exponents
-
Solution: Use the one-step method to group like terms together, and then simplify the resulting expression using exponent rules.
-
Challenge: Simplifying expressions with fractions
-
Solution: Use the one-step method to group like terms together, and then simplify the resulting expression using fraction rules.
Practical Examples
Here are some practical examples of simplifying expressions using the one-step method:
- Example 1: Simplify the expression: 3x + 2y - 2x + 4y
Solution: (3x - 2x) + (2y + 4y)
= x + 6y
- Example 2: Simplify the expression: 2x^2 + 3x - 2x^2 + 4x
Solution: (2x^2 - 2x^2) + (3x + 4x)
= 7x
Conclusion
Simplifying expressions can be a daunting task, but with the right approach, it can be done in just one easy step. The one-step method to simplify expressions involves using the concept of "grouping" to rearrange the terms of an expression and simplify it. By following this approach, you can simplify expressions quickly and accurately, and improve your problem-solving skills.
What is the one-step method to simplify expressions?
+The one-step method to simplify expressions involves using the concept of "grouping" to rearrange the terms of an expression and simplify it. This approach involves grouping like terms together and combining them.
What are the benefits of the one-step method to simplify expressions?
+The one-step method to simplify expressions offers several benefits, including reduced errors, improved understanding, and enhanced problem-solving skills.
How can I simplify expressions with multiple variables using the one-step method?
+To simplify expressions with multiple variables using the one-step method, group like terms together, and then simplify the resulting expression.
We hope this article has provided you with a comprehensive understanding of the one-step method to simplify expressions. Try using this approach to simplify expressions, and see the difference it can make in your problem-solving skills. Share your experiences and tips in the comments section below, and don't forget to share this article with your friends and colleagues.