Simplifying Square Roots: 5 Ways To Express √18

Quick Links :

When dealing with square roots, it's essential to understand how to simplify them to make calculations easier. Simplifying square roots involves expressing them in the most basic form possible, which can help in solving equations, calculating areas, and performing other mathematical operations. One such square root that can be simplified is √18. In this article, we'll explore five ways to express √18, making it easier to work with in various mathematical contexts.

Understanding Square Roots

Before diving into the simplification of √18, it's crucial to understand what square roots represent. A square root of a number is a value that, when multiplied by itself, gives the original number. For example, the square root of 16 is 4 because 4 multiplied by 4 equals 16. Square roots are denoted by the symbol √ and are used extensively in mathematics, physics, and engineering.

Importance of Simplifying Square Roots

Simplifying square roots is important for several reasons:

• Easier Calculations: Simplified square roots make mathematical calculations easier and less prone to errors.
• Clearer Understanding: Simplification helps in understanding the properties and behavior of square roots, which is crucial in advanced mathematical concepts.
• Accuracy in Measurements: In real-world applications, such as architecture and engineering, accurate calculations involving square roots are critical for safety and efficiency.

5 Ways to Express √18

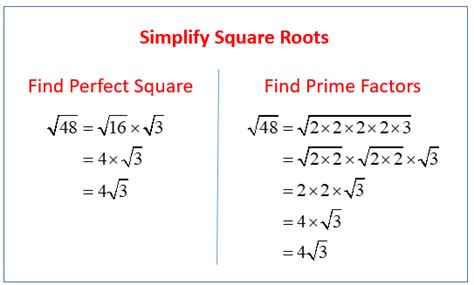
1. Using Prime Factorization

One of the most effective ways to simplify square roots is through prime factorization. This involves breaking down the number under the square root sign into its prime factors.

• Step 1: Factorize 18 into its prime factors: 18 = 2 × 3 × 3.
• Step 2: Identify the pairs of the same prime factors. In this case, we have a pair of 3s.
• Step 3: Take the square root of the pair (3 × 3) which is 3. The remaining prime factor (2) stays under the square root sign.
• Result: √18 = √(2 × 3 × 3) = 3√2.

2. Simplifying through Rationalization

Rationalizing the denominator involves getting rid of any radicals in the denominator. This method can also help in simplifying square roots when the number under the square root sign is not a perfect square.

• Step 1: Express √18 as √(18/1).
• Step 2: Rationalize the denominator by multiplying both the numerator and the denominator by √18 to get rid of the radical in the denominator.
• Result: After rationalization, we still find that the simplest form is 3√2.

3. Using Perfect Squares

Identifying perfect squares within the number under the square root sign can significantly simplify the process.

• Step 1: Identify if 18 contains a perfect square. In this case, 18 = 9 × 2, and 9 is a perfect square (3^2).
• Step 2: Take the square root of the perfect square (9) which is 3, and keep the remaining part (2) under the square root sign.
• Result: √18 = √(9 × 2) = 3√2.

4. Simplifying with Conjugates

Using conjugates is more commonly associated with rationalizing denominators but can also be applied in simplifying square roots in certain contexts.

• Step 1: Identify the conjugate of the number under the square root sign. For √18, a conjugate would be a number that, when multiplied by √18, would remove the radical. However, this method directly applies more to rationalization rather than simplification of square roots like √18.
• Result: While not directly applicable for simplification into a different form, it remains 3√2.

5. Using Mathematical Software or Calculators

In the digital age, mathematical software and calculators can instantly simplify square roots.

• Step 1: Input √18 into a calculator or mathematical software.
• Result: The output will be the simplified form of the square root, which in this case is 3√2.

Conclusion and Call to Action

Simplifying square roots like √18 is a fundamental skill in mathematics and science. By understanding and applying the methods outlined above, individuals can improve their mathematical proficiency and problem-solving abilities. Whether it's through prime factorization, rationalization, identifying perfect squares, or using digital tools, each method offers a unique perspective on simplifying square roots.

If you found this guide helpful in understanding how to simplify square roots, we encourage you to explore more mathematical topics and share your newfound knowledge with others. Mathematics is about continuous learning and application, and mastering the simplification of square roots is just one step in that journey.