The right triangle is a fundamental concept in geometry, and understanding which combinations of side lengths form a valid right triangle is crucial for various mathematical and real-world applications. In this article, we will delve into the world of right triangles, exploring the Pythagorean theorem, the different types of right triangles, and the various combinations of side lengths that work.
What is a Right Triangle?

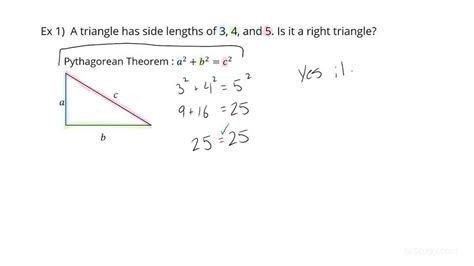
A right triangle is a triangle with one right angle (90 degrees). The side opposite the right angle is called the hypotenuse, and the other two sides are called legs. The Pythagorean theorem states that in a right triangle, the square of the length of the hypotenuse (c) is equal to the sum of the squares of the lengths of the legs (a and b): a^2 + b^2 = c^2.
The Pythagorean Theorem
The Pythagorean theorem is a fundamental concept in geometry, and it has numerous applications in mathematics, physics, engineering, and other fields. The theorem can be used to find the length of the hypotenuse of a right triangle, given the lengths of the legs, or to find the length of a leg, given the length of the hypotenuse and the other leg.
Types of Right Triangles

There are several types of right triangles, including:
- Pythagorean triples: These are right triangles with integer side lengths. Examples include 3-4-5, 5-12-13, and 8-15-17.
- Isosceles right triangles: These are right triangles with two equal legs.
- Scalene right triangles: These are right triangles with all sides of different lengths.
Combinations of Side Lengths that Work
Not all combinations of side lengths form a valid right triangle. The Pythagorean theorem can be used to determine whether a given combination of side lengths works. Here are some examples:
- 3-4-5: This is a classic Pythagorean triple, and it works because 3^2 + 4^2 = 9 + 16 = 25 = 5^2.
- 5-12-13: This is another Pythagorean triple, and it works because 5^2 + 12^2 = 25 + 144 = 169 = 13^2.
- 8-15-17: This is also a Pythagorean triple, and it works because 8^2 + 15^2 = 64 + 225 = 289 = 17^2.
On the other hand, some combinations of side lengths do not work. For example:
- 3-5-6: This combination does not work because 3^2 + 5^2 = 9 + 25 = 34, which is not equal to 6^2 = 36.
- 4-9-12: This combination does not work because 4^2 + 9^2 = 16 + 81 = 97, which is not equal to 12^2 = 144.
Real-World Applications of Right Triangles

Right triangles have numerous real-world applications, including:
- Building design: Right triangles are used in building design to ensure that walls and roofs are square and that buildings are stable.
- Physics and engineering: Right triangles are used to calculate distances, velocities, and forces in physics and engineering.
- Navigation: Right triangles are used in navigation to calculate distances and directions between locations.
Conclusion
In conclusion, right triangles are a fundamental concept in geometry, and understanding which combinations of side lengths work is crucial for various mathematical and real-world applications. The Pythagorean theorem is a powerful tool for determining whether a given combination of side lengths works, and it has numerous applications in mathematics, physics, engineering, and other fields.
We hope this article has provided you with a deeper understanding of right triangles and their applications. Whether you're a student, a teacher, or simply someone interested in mathematics, we encourage you to explore the fascinating world of right triangles further.
What is the Pythagorean theorem?
+The Pythagorean theorem states that in a right triangle, the square of the length of the hypotenuse (c) is equal to the sum of the squares of the lengths of the legs (a and b): a^2 + b^2 = c^2.
What are Pythagorean triples?
+Pythagorean triples are right triangles with integer side lengths. Examples include 3-4-5, 5-12-13, and 8-15-17.
What are some real-world applications of right triangles?
+Right triangles have numerous real-world applications, including building design, physics and engineering, and navigation.