3 Simple Forms Of A Quadratic Equation

Quick Links :

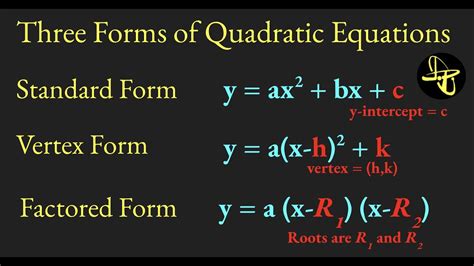
Quadratic equations are a fundamental concept in algebra and mathematics, used to describe a wide range of phenomena in physics, engineering, economics, and other fields. In its simplest form, a quadratic equation is a polynomial equation of degree two, which means the highest power of the variable (usually x) is two. The general form of a quadratic equation is ax^2 + bx + c = 0, where a, b, and c are constants. However, quadratic equations can be expressed in several forms, each with its own unique characteristics and applications. Here, we will explore three simple forms of a quadratic equation.

Standard Form: ax^2 + bx + c = 0

The standard form of a quadratic equation is the most common and widely used form. It is expressed as ax^2 + bx + c = 0, where a, b, and c are constants, and a cannot be zero. This form is useful for factoring, solving, and analyzing quadratic equations. For example, the equation x^2 + 5x + 6 = 0 is in standard form, where a = 1, b = 5, and c = 6.

Factored Form: (x - p)(x - q) = 0

The factored form of a quadratic equation is a product of two binomials, where each binomial contains one of the roots of the equation. This form is useful for finding the roots or solutions of a quadratic equation. For example, the equation (x - 2)(x - 3) = 0 is in factored form, where p = 2 and q = 3 are the roots of the equation.

Vertex Form: a(x - h)^2 + k = 0

The vertex form of a quadratic equation is a special form that provides information about the vertex of the parabola. This form is useful for graphing and analyzing quadratic equations. For example, the equation (x - 1)^2 + 2 = 0 is in vertex form, where h = 1 and k = 2 are the coordinates of the vertex.

Benefits of Using Different Forms

Using different forms of a quadratic equation can provide various benefits, such as:

• Simplifying complex equations
• Identifying roots and solutions
• Graphing and analyzing parabolas
• Solving problems in physics, engineering, and other fields

By understanding the different forms of a quadratic equation, you can choose the most suitable form for a particular problem or application.

Real-World Applications

Quadratic equations have numerous real-world applications in physics, engineering, economics, and other fields. For example:

• Projectile motion: Quadratic equations can be used to model the trajectory of a projectile, such as a ball or a rocket.
• Electrical circuits: Quadratic equations can be used to analyze and design electrical circuits, such as filters and amplifiers.
• Finance: Quadratic equations can be used to model and analyze financial systems, such as stock prices and interest rates.

By understanding the different forms of a quadratic equation, you can apply them to a wide range of real-world problems and applications.

Conclusion

In conclusion, quadratic equations are a fundamental concept in algebra and mathematics, with various forms and applications. By understanding the standard, factored, and vertex forms of a quadratic equation, you can simplify complex equations, identify roots and solutions, graph and analyze parabolas, and solve problems in physics, engineering, and other fields. Whether you are a student, teacher, or professional, mastering quadratic equations can provide a solid foundation for advanced mathematics and real-world applications.

Now, take a moment to share your thoughts and questions about quadratic equations in the comments below. How do you use quadratic equations in your studies or work? What are some common challenges or misconceptions about quadratic equations?