Radical expressions, also known as roots, are a fundamental concept in mathematics that can seem daunting at first, but with practice and the right techniques, they can become second nature. Radical expressions are used to represent a number that, when multiplied by itself, gives a specified value. In this article, we will explore the world of radical expressions, learn how to simplify them, and discover the techniques to make them easier to work with.
What are Radical Expressions?

Radical expressions are mathematical expressions that contain a radical sign, which is denoted by the symbol √. The radical sign indicates that the number inside the sign is being "taken out" or "extracted" from under the radical sign. For example, √16 means "the square root of 16," which is the number that, when multiplied by itself, gives 16. In this case, the square root of 16 is 4, since 4 × 4 = 16.
Why Simplify Radical Expressions?

Simplifying radical expressions is essential in mathematics because it helps to:
- Reduce the complexity of expressions
- Make calculations easier
- Identify equivalent expressions
- Solve equations and inequalities
By simplifying radical expressions, you can make them more manageable and easier to work with, which is crucial in various mathematical applications.
Basic Rules for Simplifying Radical Expressions
To simplify radical expressions, follow these basic rules:
- Rule 1: √(a × b) = √a × √b
- Rule 2: √(a/b) = √a / √b
- Rule 3: If a is a perfect square, then √a = a
- Rule 4: If a is not a perfect square, then √a cannot be simplified further
These rules will help you to simplify radical expressions and make them more manageable.
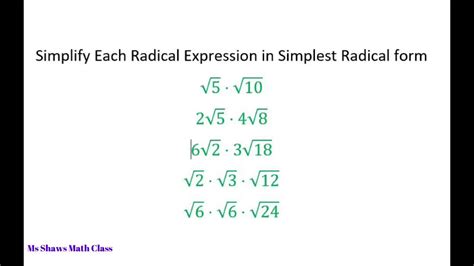
Examples of Simplifying Radical Expressions

Let's consider some examples of simplifying radical expressions:
- √(16 × 4) = √16 × √4 = 4 × 2 = 8
- √(9/4) = √9 / √4 = 3 / 2
- √25 = 5 (since 25 is a perfect square)
- √10 cannot be simplified further (since 10 is not a perfect square)
By applying the basic rules, you can simplify radical expressions and make them easier to work with.
Advanced Techniques for Simplifying Radical Expressions

In addition to the basic rules, there are advanced techniques that can help you simplify radical expressions:
- Rationalizing the denominator: Multiply the numerator and denominator by the conjugate of the denominator to eliminate the radical sign in the denominator.
- Simplifying cube roots: Use the formula ∛(a × b) = ∛a × ∛b to simplify cube roots.
- Simplifying nth roots: Use the formula ∜(a × b) = ∜a × ∜b to simplify nth roots.
These advanced techniques will help you to simplify more complex radical expressions and make them more manageable.
Practical Applications of Simplifying Radical Expressions
Simplifying radical expressions has numerous practical applications in various fields, including:
- Physics: Simplifying radical expressions is essential in physics to solve equations and inequalities that describe the behavior of physical systems.
- Engineering: Simplifying radical expressions is crucial in engineering to design and optimize systems, such as electronic circuits and mechanical systems.
- Computer Science: Simplifying radical expressions is used in computer science to develop algorithms and solve problems in computer graphics and game development.
By simplifying radical expressions, you can make complex calculations easier and more manageable, which is essential in various practical applications.
Conclusion

In conclusion, simplifying radical expressions is a crucial skill in mathematics that can seem daunting at first, but with practice and the right techniques, it can become second nature. By mastering the basic rules and advanced techniques, you can simplify radical expressions and make them more manageable, which is essential in various mathematical applications.
We hope this article has helped you to understand the importance of simplifying radical expressions and how to do it effectively. If you have any questions or comments, please feel free to share them below.
What is the purpose of simplifying radical expressions?
+The purpose of simplifying radical expressions is to reduce the complexity of expressions, make calculations easier, identify equivalent expressions, and solve equations and inequalities.
What are the basic rules for simplifying radical expressions?
+The basic rules for simplifying radical expressions are:
- √(a × b) = √a × √b
- √(a/b) = √a / √b
- If a is a perfect square, then √a = a
- If a is not a perfect square, then √a cannot be simplified further
What are some practical applications of simplifying radical expressions?
+Simplifying radical expressions has numerous practical applications in various fields, including physics, engineering, and computer science.