Simplify 28 To A Fraction In 1 Easy Step

Quick Links :

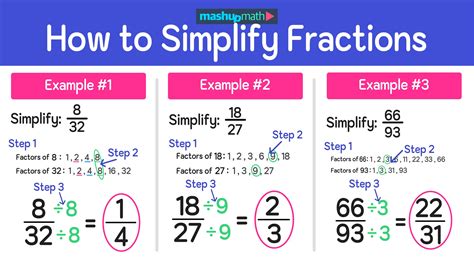
28 can be simplified to a fraction by dividing it by 1. Since 28 is a whole number, it can be expressed as a fraction with a denominator of 1.

Simplifying 28 to a Fraction

28 = 28/1

This fraction cannot be simplified further, as the greatest common divisor (GCD) of 28 and 1 is 1.

Why Simplify Numbers to Fractions?

Simplifying numbers to fractions is essential in mathematics, especially when dealing with decimals, percentages, or ratios. By expressing a number as a fraction, you can:

• Identify equivalent ratios and proportions
• Compare and order fractions
• Perform arithmetic operations, such as addition and subtraction, with fractions
• Convert between fractions, decimals, and percentages

In the next section, we will explore more about fractions and their importance in mathematics.

What are Fractions?

A fraction is a way to represent a part of a whole. It consists of two numbers: the numerator (the top number) and the denominator (the bottom number). The numerator tells us how many equal parts we have, while the denominator tells us how many parts the whole is divided into.

For example, the fraction 1/2 represents one equal part out of a total of two parts.

Types of Fractions

There are different types of fractions, including:

• Proper fractions: The numerator is less than the denominator, e.g., 1/2 or 3/4.
• Improper fractions: The numerator is greater than or equal to the denominator, e.g., 3/2 or 5/3.
• Mixed numbers: A combination of a whole number and a proper fraction, e.g., 2 1/2 or 3 3/4.

In the next section, we will discuss how to add and subtract fractions.

Adding and Subtracting Fractions

To add or subtract fractions, we need to follow these steps:

• Ensure the fractions have the same denominator
• Add or subtract the numerators
• Keep the denominator the same

For example, let's add 1/4 and 1/4:

1/4 + 1/4 = 2/4

Since the denominators are the same, we can add the numerators (1 + 1 = 2) and keep the denominator the same (4).

Real-World Applications of Fractions

Fractions are used in various real-world scenarios, such as:

• Cooking and recipes
• Measuring ingredients and quantities
• Understanding music and rhythm
• Analyzing data and statistics

In the next section, we will explore how to multiply and divide fractions.

Multiplying and Dividing Fractions

To multiply fractions, we multiply the numerators and multiply the denominators.

For example, let's multiply 1/2 and 3/4:

(1/2) × (3/4) = (1 × 3) / (2 × 4) = 3/8

To divide fractions, we invert the second fraction (i.e., flip the numerator and denominator) and multiply.

For example, let's divide 1/2 by 3/4:

(1/2) ÷ (3/4) = (1/2) × (4/3) = 4/6

Common Challenges with Fractions

Some common challenges when working with fractions include:

• Understanding equivalent ratios and proportions
• Simplifying complex fractions
• Performing arithmetic operations with fractions

In the next section, we will discuss how to overcome these challenges.

Overcoming Challenges with Fractions

To overcome challenges with fractions, try the following strategies:

• Practice, practice, practice: The more you work with fractions, the more comfortable you will become.
• Use visual aids: Draw diagrams or pictures to help you understand equivalent ratios and proportions.
• Break problems down: Simplify complex fractions by breaking them down into smaller parts.

By following these tips and practicing regularly, you can become proficient in working with fractions.

Conclusion

In this article, we have explored the world of fractions, from simplifying numbers to fractions to performing arithmetic operations. We have also discussed real-world applications of fractions and common challenges that arise when working with them.

By mastering fractions, you can improve your mathematical skills and become more confident in your ability to tackle complex problems.

We hope you have enjoyed this article and found it informative. If you have any questions or comments, please feel free to share them below.