Factoring Quadratic Expressions: 4x^2 + 12x + 5

Quick Links :

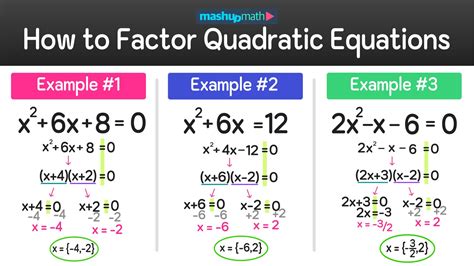
Quadratic expressions are a fundamental part of algebra, and factoring them is an essential skill for any math student. In this article, we will delve into the world of factoring quadratic expressions, using the example of 4x^2 + 12x + 5. By the end of this article, you will have a solid understanding of how to factor quadratic expressions and be able to apply this knowledge to a wide range of problems.

First, let's start with the basics. A quadratic expression is a polynomial of degree two, which means it can be written in the form ax^2 + bx + c, where a, b, and c are constants. Factoring a quadratic expression involves expressing it as the product of two binomials. In other words, we want to rewrite the expression in the form (px + q)(rx + s), where p, q, r, and s are constants.

Factoring Quadratic Expressions: Benefits and Applications

Factoring quadratic expressions is an important skill in algebra, and it has many real-world applications. By factoring quadratic expressions, we can:

• Simplify complex expressions and make them easier to work with
• Solve quadratic equations and find the roots of the equation
• Find the x-intercepts of a quadratic function
• Determine the vertex of a quadratic function

Factoring Quadratic Expressions: Methods and Techniques

There are several methods and techniques for factoring quadratic expressions. In this section, we will cover the most common methods.

Method 1: Factoring by Greatest Common Factor (GCF)

The first method we will cover is factoring by greatest common factor (GCF). This method involves finding the GCF of the terms in the quadratic expression and factoring it out.

For example, let's consider the quadratic expression 6x^2 + 12x + 6. The GCF of the terms is 6, so we can factor it out as follows:

6x^2 + 12x + 6 = 6(x^2 + 2x + 1)

As you can see, this method is useful when the quadratic expression has a common factor among all the terms.

Method 2: Factoring by Grouping

The second method we will cover is factoring by grouping. This method involves grouping the terms in the quadratic expression and factoring out a common factor from each group.

For example, let's consider the quadratic expression x^2 + 5x + 6. We can group the terms as follows:

x^2 + 5x + 6 = (x^2 + 3x) + (2x + 6)

Now, we can factor out a common factor from each group:

x^2 + 5x + 6 = x(x + 3) + 2(x + 3)

As you can see, this method is useful when the quadratic expression can be grouped into two or more sets of terms with common factors.

Method 3: Factoring by Using the FOIL Method

The third method we will cover is factoring by using the FOIL method. This method involves using the FOIL (First, Outer, Inner, Last) method to multiply two binomials and then factoring the resulting expression.

For example, let's consider the quadratic expression x^2 + 5x + 6. We can use the FOIL method to multiply two binomials as follows:

x^2 + 5x + 6 = (x + 2)(x + 3)

As you can see, this method is useful when the quadratic expression can be factored into the product of two binomials.

Factoring Quadratic Expressions: Steps and Examples

Now that we have covered the methods and techniques for factoring quadratic expressions, let's move on to the steps and examples.

Step 1: Write the Quadratic Expression

The first step is to write the quadratic expression in the form ax^2 + bx + c.

Step 2: Factor Out the Greatest Common Factor (GCF)

The second step is to factor out the GCF of the terms in the quadratic expression.

Step 3: Factor the Remaining Terms

The third step is to factor the remaining terms using one of the methods we covered earlier (factoring by grouping, factoring by using the FOIL method, etc.).

Let's consider an example. Suppose we want to factor the quadratic expression 4x^2 + 12x + 5. We can follow the steps as follows:

Step 1: Write the Quadratic Expression

4x^2 + 12x + 5

Step 2: Factor Out the Greatest Common Factor (GCF)

The GCF of the terms is 1, so we cannot factor out a common factor.

Step 3: Factor the Remaining Terms

We can use the FOIL method to factor the remaining terms as follows:

4x^2 + 12x + 5 = (2x + 1)(2x + 5)

As you can see, the factored form of the quadratic expression is (2x + 1)(2x + 5).

Common Mistakes to Avoid When Factoring Quadratic Expressions

When factoring quadratic expressions, there are several common mistakes to avoid. Here are a few:

• Not factoring out the GCF: Make sure to factor out the GCF of the terms in the quadratic expression.
• Not using the correct method: Make sure to use the correct method for factoring the quadratic expression (factoring by grouping, factoring by using the FOIL method, etc.).
• Not checking the factored form: Make sure to check the factored form of the quadratic expression to ensure it is correct.

Frequently Asked Questions (FAQs)

Q: What is factoring a quadratic expression?

A: Factoring a quadratic expression involves expressing it as the product of two binomials.

Q: What are the methods for factoring quadratic expressions?

A: There are several methods for factoring quadratic expressions, including factoring by greatest common factor (GCF), factoring by grouping, and factoring by using the FOIL method.

Q: How do I know which method to use?

A: The method you use will depend on the specific quadratic expression you are trying to factor. You may need to try different methods to find the one that works best.

Conclusion and Final Thoughts

In conclusion, factoring quadratic expressions is an essential skill in algebra. By understanding the methods and techniques for factoring quadratic expressions, you can simplify complex expressions, solve quadratic equations, and find the x-intercepts of a quadratic function. Remember to avoid common mistakes, such as not factoring out the GCF or not using the correct method. With practice and patience, you can master the art of factoring quadratic expressions.