Simplifying 3 18: Easy Steps To Reduce Fractions

Quick Links :

Simplifying fractions is a fundamental math concept that can be a bit tricky, but with the right approach, it can be made easy. Reducing fractions to their simplest form is an essential skill that can help you solve various math problems with ease. In this article, we will explore the steps to simplify fractions and provide practical examples to help you master this concept.

Understanding Fractions

Before we dive into simplifying fractions, let's quickly review what fractions are. A fraction is a way to represent a part of a whole. It consists of two numbers: the numerator (the top number) and the denominator (the bottom number). For example, in the fraction 3/4, 3 is the numerator, and 4 is the denominator.

Why Simplify Fractions?

Simplifying fractions is essential for several reasons:

• It makes math problems easier to solve.
• It helps to avoid confusion when working with fractions.
• It is a crucial step in more advanced math concepts, such as algebra and calculus.

Step-by-Step Guide to Simplifying Fractions

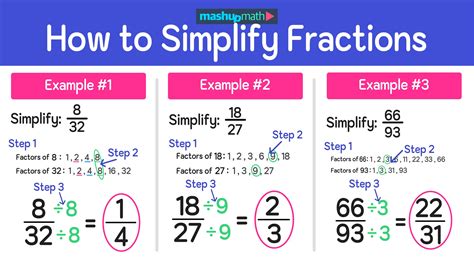
Simplifying fractions involves dividing both the numerator and the denominator by the greatest common divisor (GCD). Here are the easy steps to follow:

Step 1: Find the Greatest Common Divisor (GCD)

The first step in simplifying a fraction is to find the greatest common divisor (GCD) of the numerator and the denominator. The GCD is the largest number that divides both numbers without leaving a remainder.

Step 2: Divide the Numerator and Denominator by the GCD

Once you have found the GCD, divide both the numerator and the denominator by the GCD. This will give you the simplified fraction.

Step 3: Check if the Fraction is in its Simplest Form

After simplifying the fraction, check if it is in its simplest form. If the numerator and the denominator have a common factor, you may need to simplify the fraction further.

Example: Simplifying a Fraction

Let's use the fraction 12/18 as an example.

Step 1: Find the Greatest Common Divisor (GCD)

The GCD of 12 and 18 is 6.

Step 2: Divide the Numerator and Denominator by the GCD

Divide both the numerator and the denominator by 6:

12 ÷ 6 = 2 18 ÷ 6 = 3

So, the simplified fraction is 2/3.

Step 3: Check if the Fraction is in its Simplest Form

The fraction 2/3 is in its simplest form, as the numerator and the denominator have no common factors.

Common Mistakes to Avoid

When simplifying fractions, there are a few common mistakes to avoid:

• Not finding the GCD: Make sure to find the GCD of the numerator and the denominator before simplifying the fraction.
• Dividing by the wrong number: Double-check that you are dividing both the numerator and the denominator by the correct number (the GCD).
• Not checking if the fraction is in its simplest form: Always check if the fraction is in its simplest form after simplifying it.

Tips and Tricks

Here are a few tips and tricks to help you simplify fractions with ease:

• Use a calculator: If you are struggling to find the GCD or simplify the fraction, use a calculator to help you.
• Practice, practice, practice: The more you practice simplifying fractions, the easier it will become.
• Break down complex fractions: If you are working with complex fractions, break them down into simpler fractions to make it easier to simplify.

Real-World Applications

Simplifying fractions has many real-world applications:

• Cooking: When following a recipe, you may need to simplify fractions to measure ingredients correctly.
• Finance: Simplifying fractions is essential when working with interest rates, investments, and other financial calculations.
• Science: Fractions are used extensively in science, and simplifying them is crucial for accurate calculations.

Conclusion

Simplifying fractions is a fundamental math concept that can be made easy with the right approach. By following the easy steps outlined in this article, you can simplify fractions with ease and master this essential math skill. Remember to practice regularly and use the tips and tricks provided to help you become a pro at simplifying fractions.