75 As A Fraction: Simplified Math Explained

Quick Links :

The concept of fractions is a fundamental aspect of mathematics, and understanding how to work with them is crucial for success in various fields. One common fraction that people often struggle with is 75 as a fraction. In this article, we will break down the concept of 75 as a fraction, explain how to simplify it, and provide examples and practical applications.

What is a Fraction?

A fraction is a way to represent a part of a whole. It consists of two numbers: the numerator and the denominator. The numerator tells us how many equal parts we have, and the denominator tells us how many parts the whole is divided into. For example, the fraction 1/2 represents one equal part out of a total of two parts.

75 as a Fraction

So, how can we represent 75 as a fraction? The simplest way to do this is to write it as 75/1. This tells us that we have 75 equal parts, and the whole is divided into one part. However, this fraction can be simplified further.

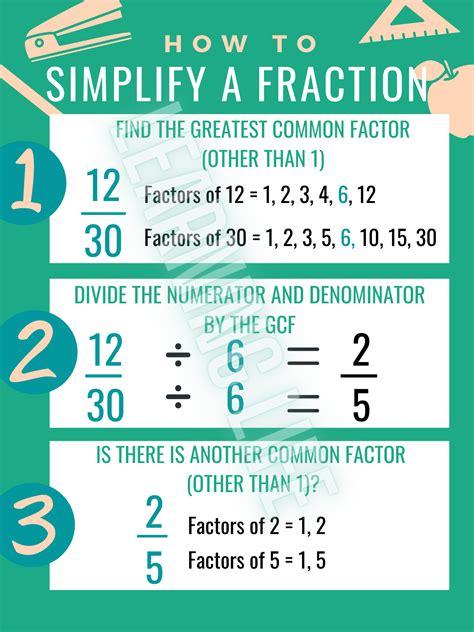
Simplifying Fractions

To simplify a fraction, we need to find the greatest common divisor (GCD) of the numerator and denominator. The GCD is the largest number that divides both the numerator and denominator without leaving a remainder. Once we find the GCD, we can divide both the numerator and denominator by this number to simplify the fraction.

In the case of 75/1, the GCD is 1, since 1 is the only number that divides both 75 and 1 without leaving a remainder. Therefore, the fraction 75/1 is already in its simplest form.

75 as a Fraction with Different Denominators

However, we can represent 75 as a fraction with different denominators. For example, we can write 75 as 150/2 or 225/3. These fractions are equivalent to 75/1, but they have different denominators.

Practical Applications of 75 as a Fraction

Now that we understand how to represent 75 as a fraction, let's explore some practical applications. Fractions are commonly used in cooking, measurement, and finance. For example, if a recipe calls for 3/4 cup of sugar, and you only have 1/2 cup, you can use the fraction 75/100 to calculate the equivalent amount.

Fraction to Percentage Conversion

Another important concept related to fractions is percentage conversion. To convert a fraction to a percentage, we need to divide the numerator by the denominator and multiply by 100.

For example, to convert the fraction 75/100 to a percentage, we can divide 75 by 100 and multiply by 100, which gives us 75%.

Real-World Examples of 75 as a Fraction

Let's consider some real-world examples of 75 as a fraction. Suppose you have a bag of 75 apples, and you want to divide them equally among 3 people. You can represent this as the fraction 75/3, which simplifies to 25/1.

Another example is a company that has 75 employees, and 25 of them are managers. We can represent this as the fraction 25/75, which simplifies to 1/3.

Common Mistakes When Working with 75 as a Fraction

When working with fractions, it's easy to make mistakes. Here are some common errors to watch out for:

• Not simplifying fractions correctly
• Not finding the greatest common divisor (GCD) correctly
• Not converting fractions to percentages correctly

To avoid these mistakes, make sure to double-check your calculations and use online resources or calculators to verify your answers.

Tips for Working with 75 as a Fraction

Here are some tips for working with 75 as a fraction:

• Simplify fractions whenever possible
• Use equivalent fractions to compare values
• Convert fractions to percentages to make comparisons easier
• Use real-world examples to practice working with fractions

H2: Fraction Operations

Adding and Subtracting Fractions

To add or subtract fractions, we need to have the same denominator. We can do this by finding the least common multiple (LCM) of the denominators.

For example, to add 1/2 and 1/4, we can find the LCM of 2 and 4, which is 4. Then, we can rewrite the fractions with the same denominator:

1/2 = 2/4 1/4 = 1/4

Now, we can add the fractions:

2/4 + 1/4 = 3/4

Multiplying and Dividing Fractions

To multiply fractions, we can multiply the numerators and denominators separately.

For example, to multiply 1/2 and 3/4, we can multiply the numerators and denominators:

1/2 × 3/4 = 3/8

To divide fractions, we can invert the second fraction (i.e., flip the numerator and denominator) and multiply.

For example, to divide 1/2 by 3/4, we can invert the second fraction and multiply:

1/2 ÷ 3/4 = 1/2 × 4/3 = 4/6

H3: Real-World Applications of Fraction Operations

Fraction operations are used in various real-world applications, such as cooking, measurement, and finance. For example, if a recipe calls for 3/4 cup of sugar, and you only have 1/2 cup, you can use fraction operations to calculate the equivalent amount.

H2: Conclusion

In conclusion, 75 as a fraction is a simple concept that can be represented in various ways. By understanding how to simplify fractions, convert them to percentages, and perform fraction operations, we can tackle real-world problems with confidence.

Whether you're a student, teacher, or professional, mastering fractions is essential for success in various fields. We hope this article has provided you with a deeper understanding of 75 as a fraction and how to work with it.

FAQ Section