Simplify 25 Into Fraction Form In 1 Easy Step

Quick Links :

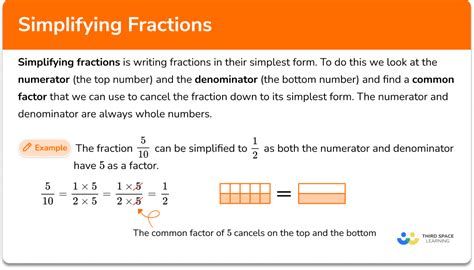
Simplifying numbers into fraction form can often seem like a daunting task, but it's actually quite straightforward. The key to simplifying a number like 25 into a fraction is to understand what fractions represent - a part of a whole.

25, as a standalone number, is not a fraction. However, it can be expressed as a fraction in its simplest form. To do this, you simply divide 25 by 1, since any number divided by 1 is itself. This might seem like a trivial step, but it's how you represent a whole number as a fraction.

Step to Simplify 25 into Fraction Form

What does Simplifying Mean?

Simplifying a number into a fraction means expressing it in its most reduced form. However, for a number like 25, which is a whole number, its simplest fraction form would just be 25/1.

The step is as follows:

1. Express 25 as a fraction by dividing it by 1. So, the fraction form of 25 is 25/1.

And that's it! You've successfully simplified 25 into its fraction form in one easy step.

Understanding the Concept of Fractions

Fractions represent a part of a whole. They consist of two main parts: the numerator (the number on top) and the denominator (the number at the bottom). The numerator tells you how many equal parts you have, and the denominator tells you how many parts the whole is divided into.

In the case of 25/1, the whole is divided into 1 part, and you have 25 of that part. Essentially, it means you have the whole thing 25 times, which is why it's still just 25.

Real-World Applications of Simplifying Numbers

While simplifying 25 into a fraction might seem trivial, understanding how to express numbers in fraction form is crucial in various real-world applications. From cooking to construction, fractions help us understand proportions and measurements more accurately.

For instance, a recipe might call for 1/4 teaspoon of a spice. If you're making a batch that's four times the recipe, you'll need 1 teaspoon (4 * 1/4). This shows how fractions can help in scaling up or down.

Common Challenges and Misconceptions

One common misconception about fractions is that they're always less than one. However, as we've seen with 25/1, fractions can represent whole numbers or even multiples of whole numbers.

Another challenge people face is simplifying fractions. To simplify a fraction, you find the greatest common divisor (GCD) of the numerator and the denominator and divide both by this number. However, in the case of 25/1, the fraction is already in its simplest form because 1 is the smallest possible denominator.

Practical Examples of Simplifying Fractions

Let's take a fraction like 10/2. To simplify this, you find the GCD of 10 and 2, which is 2. Dividing both the numerator and the denominator by 2 gives you 5/1, which simplifies to just 5.

Conclusion: Simplification into Practice

Simplifying numbers into fraction form is a straightforward process, even if the number is a whole number like 25. Understanding fractions and how to simplify them is crucial for everyday applications and for advancing in mathematical studies.

Whether you're working with fractions in a recipe, a science experiment, or a mathematical equation, knowing how to simplify them can help you solve problems more efficiently.

By understanding and applying these concepts, you can enhance your mathematical skills and tackle more complex problems with confidence.

Final Thoughts

Simplifying numbers like 25 into fraction form might seem simple, but it underscores a fundamental concept in mathematics: the representation of whole numbers and parts thereof. Whether you're a student or someone looking to improve your math skills, practicing how to simplify fractions can make a significant difference in your problem-solving abilities.