Supplementary angles are a fundamental concept in geometry, and their relationship with linear pairs is a crucial aspect of understanding angles and their properties. In this article, we will delve into the world of supplementary angles and linear pairs, exploring their definitions, properties, and applications.
What are Supplementary Angles?

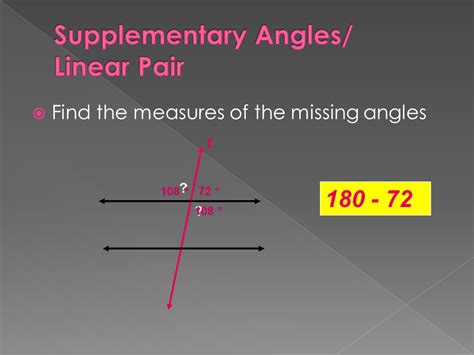
Supplementary angles are two angles whose measures add up to 180 degrees. In other words, if we have two angles, A and B, and their sum is 180 degrees, then they are supplementary angles. This concept is often represented as:
∠A + ∠B = 180°
where ∠A and ∠B are the measures of the two angles.
Properties of Supplementary Angles
Supplementary angles have several important properties:
- The sum of the measures of two supplementary angles is always 180 degrees.
- If two angles are supplementary, then their sum is equal to the sum of the measures of two right angles (90 degrees each).
- If two angles are supplementary, then one angle is not necessarily equal to the other.
What is a Linear Pair?

A linear pair is a pair of adjacent angles that form a straight line. In other words, two angles that share a common vertex (corner point) and have no overlap between them form a linear pair. The measures of the angles in a linear pair add up to 180 degrees.
Properties of Linear Pairs
Linear pairs have several important properties:
- The sum of the measures of the angles in a linear pair is always 180 degrees.
- The angles in a linear pair are always supplementary.
- Linear pairs are also known as adjacent angles.
Relationship between Supplementary Angles and Linear Pairs

There is a deep connection between supplementary angles and linear pairs. In fact, supplementary angles form a linear pair. This means that if we have two supplementary angles, they must form a straight line. Conversely, if we have a linear pair, then the angles are supplementary.
This relationship can be represented as:
∠A + ∠B = 180°
where ∠A and ∠B are the measures of the angles in the linear pair.
Examples and Applications
Supplementary angles and linear pairs have numerous applications in various fields, including architecture, engineering, and design. For example:
- In building design, architects use supplementary angles to create aesthetically pleasing and functional spaces.
- In engineering, supplementary angles are used to design stable and efficient structures.
- In art and design, supplementary angles are used to create visually appealing compositions.
Real-World Examples

Here are some real-world examples of supplementary angles and linear pairs:
- A door hinge forms a linear pair with the door frame.
- A bookshelf forms a linear pair with the wall.
- A bridge forms a linear pair with the riverbank.
Conclusion
Supplementary angles and linear pairs are fundamental concepts in geometry that have numerous applications in various fields. Understanding the relationship between these two concepts can help us solve problems and create innovative solutions. Whether in art, design, or engineering, supplementary angles and linear pairs play a crucial role in shaping our world.

We encourage you to share your thoughts and examples of supplementary angles and linear pairs in the comments section below. How have you applied these concepts in your daily life or work?
What is the difference between supplementary angles and linear pairs?
+Supplementary angles are two angles whose measures add up to 180 degrees, while a linear pair is a pair of adjacent angles that form a straight line. All linear pairs are supplementary angles, but not all supplementary angles are linear pairs.
Can two supplementary angles be adjacent?
+Yes, two supplementary angles can be adjacent. In fact, this is the definition of a linear pair.
Can a linear pair have more than two angles?
+No, a linear pair by definition consists of only two angles.