0.125 In Fraction Form Simplified

Quick Links :

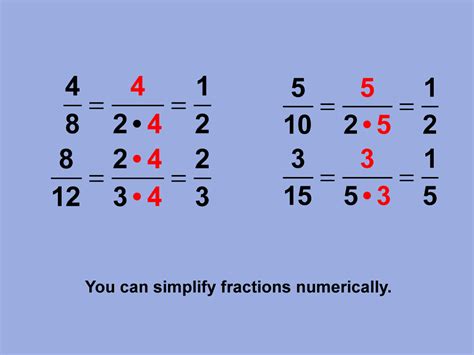
Simplifying fractions is a fundamental concept in mathematics that helps us understand and work with numerical values more efficiently. In this article, we will delve into the world of fractions, focusing on how to simplify them, and specifically, we will look at the fraction 0.125 in its simplified form.

Understanding Fractions

Before we dive into simplifying fractions, it's essential to understand what a fraction is. A fraction is a way to represent a part of a whole. It consists of two parts: the numerator (the top number) and the denominator (the bottom number). The numerator tells us how many equal parts we have, and the denominator tells us how many parts the whole is divided into.

Why Simplify Fractions?

Simplifying fractions is crucial for various mathematical operations and real-world applications. When fractions are in their simplest form, they are easier to compare, add, subtract, multiply, and divide. Simplifying fractions also helps in reducing errors and making calculations more efficient.

Simplifying Fractions

To simplify a fraction, we need to find the greatest common divisor (GCD) of the numerator and the denominator. The GCD is the largest number that divides both the numerator and the denominator without leaving a remainder. Once we find the GCD, we can divide both the numerator and the denominator by the GCD to simplify the fraction.

Converting Decimals to Fractions

The fraction 0.125 is actually a decimal value. To convert a decimal to a fraction, we can use the following steps:

1. Write the decimal as a fraction with the decimal value as the numerator and 1 as the denominator.
2. Multiply the numerator and the denominator by 10 for each decimal place.

For example, to convert 0.125 to a fraction:

0.125 = 125/1000

Simplifying 0.125

Now that we have the fraction 125/1000, we can simplify it by finding the GCD of 125 and 1000.

The factors of 125 are: 1, 5, 25, 125 The factors of 1000 are: 1, 2, 4, 5, 8, 10, 20, 25, 40, 50, 100, 125, 200, 250, 500, 1000

The greatest common divisor of 125 and 1000 is 125.

Now, we can simplify the fraction:

125/1000 = (125 ÷ 125) / (1000 ÷ 125) = 1/8

The Simplified Form of 0.125

So, the simplified form of 0.125 is 1/8.

Real-World Applications

Simplifying fractions has numerous real-world applications. For instance, in cooking, recipes often involve fractions. Simplifying these fractions can help with meal planning and ingredient measurement. In science, fractions are used to represent proportions and ratios. Simplifying fractions can make it easier to analyze and compare data.

Tips for Simplifying Fractions

Here are some tips to keep in mind when simplifying fractions:

• Always find the greatest common divisor (GCD) of the numerator and the denominator.
• Divide both the numerator and the denominator by the GCD to simplify the fraction.
• Simplify fractions before performing mathematical operations to avoid errors.
• Use visual aids, such as diagrams or charts, to help with simplification.

Conclusion

Simplifying fractions is an essential math concept that can make a significant difference in our daily lives. By understanding how to simplify fractions, we can perform mathematical operations more efficiently and accurately. The fraction 0.125, when simplified, becomes 1/8, making it easier to work with and compare. Remember to always simplify fractions before performing mathematical operations, and use visual aids to help with the process.

Frequently Asked Questions