Convert Vertex To Standard Form In 3 Easy Steps

Quick Links :

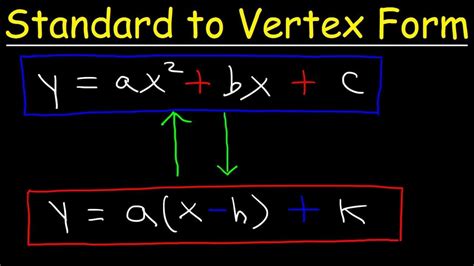
The vertex form of a quadratic function is a powerful tool for graphing and analyzing parabolas. However, sometimes it's necessary to convert the vertex form to the standard form of a quadratic function. In this article, we'll explore the process of converting vertex form to standard form in 3 easy steps.

Converting between different forms of quadratic functions can seem daunting, but with the right steps, it can be a straightforward process. Understanding how to convert vertex form to standard form can help you solve problems more efficiently and make it easier to work with quadratic functions in various mathematical contexts.

The vertex form of a quadratic function is written as f(x) = a(x - h)^2 + k, where (h, k) is the vertex of the parabola. The standard form of a quadratic function, on the other hand, is written as f(x) = ax^2 + bx + c. In this article, we'll break down the process of converting vertex form to standard form into 3 easy steps.

Step 1: Expand the Vertex Form

The first step in converting vertex form to standard form is to expand the squared term in the vertex form. To do this, we'll use the formula (x - h)^2 = x^2 - 2hx + h^2. This will allow us to rewrite the vertex form as f(x) = a(x^2 - 2hx + h^2) + k.

For example, let's say we have the vertex form f(x) = 2(x - 3)^2 + 4. We can expand the squared term as follows:

f(x) = 2(x^2 - 6x + 9) + 4

This expanded form is a crucial step in converting the vertex form to standard form.

Step 2: Distribute the Coefficient

The next step is to distribute the coefficient "a" to the terms inside the parentheses. This will allow us to rewrite the expression in a more standard form.

Using the example from Step 1, we can distribute the coefficient 2 as follows:

f(x) = 2x^2 - 12x + 18 + 4

This step helps us to simplify the expression and move closer to the standard form.

Step 3: Combine Like Terms

The final step is to combine like terms to simplify the expression. This involves adding or subtracting the coefficients of the same terms.

Using the example from Step 2, we can combine like terms as follows:

f(x) = 2x^2 - 12x + 22

This is the standard form of the quadratic function.

Why Converting Vertex Form to Standard Form is Important

Converting vertex form to standard form is an essential skill in mathematics, particularly when working with quadratic functions. Here are a few reasons why:

• Solving Equations: Standard form is often more convenient when solving quadratic equations, as it allows us to use techniques like factoring and the quadratic formula.
• Graphing: While vertex form is useful for graphing parabolas, standard form can provide additional information about the graph, such as the axis of symmetry and the x-intercepts.
• Real-World Applications: Quadratic functions are used to model real-world phenomena, such as projectile motion and population growth. Converting vertex form to standard form can help us to better understand and analyze these models.

Real-World Example

A company produces and sells a product, and the profit function is given by the vertex form P(x) = 2(x - 5)^2 + 10, where x is the number of units produced and sold. To determine the maximum profit, we need to convert the vertex form to standard form.

Using the steps outlined above, we can expand the vertex form, distribute the coefficient, and combine like terms to get the standard form:

P(x) = 2x^2 - 20x + 30

This standard form allows us to easily identify the maximum profit, which occurs when x = 5.

Common Mistakes to Avoid

When converting vertex form to standard form, there are a few common mistakes to avoid:

• Forgetting to Expand the Squared Term: Make sure to expand the squared term in the vertex form, as this is a crucial step in the conversion process.
• Distributing the Coefficient Incorrectly: Double-check that you're distributing the coefficient correctly, as this can affect the accuracy of the conversion.
• Not Combining Like Terms: Make sure to combine like terms to simplify the expression, as this will help you to get the standard form correct.

By following the 3 easy steps outlined in this article, you can confidently convert vertex form to standard form and take your quadratic function skills to the next level.

Conclusion

In conclusion, converting vertex form to standard form is a valuable skill in mathematics that can help you to better understand and work with quadratic functions. By following the 3 easy steps outlined in this article, you can convert vertex form to standard form with confidence and accuracy. Remember to avoid common mistakes, such as forgetting to expand the squared term and not combining like terms. With practice and patience, you'll become a pro at converting vertex form to standard form in no time!

FAQ: