Mathway Vertex Form Made Easy

Quick Links :

Understanding vertex form can be a game-changer for anyone working with quadratic equations and functions. Mathway, a popular online math problem solver, can be a valuable tool in making sense of this complex concept. In this article, we will delve into the world of vertex form, explore its importance, and discuss how Mathway can help make it more accessible.

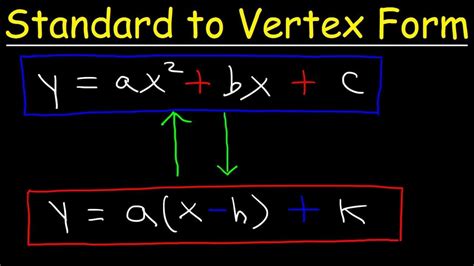
Vertex form is a way of expressing quadratic functions in a specific format, which can be incredibly useful for graphing and analyzing these functions. A quadratic function in vertex form is written as f(x) = a(x - h)^2 + k, where (h, k) represents the vertex of the parabola. This form makes it easy to identify key features of the parabola, such as its vertex, axis of symmetry, and direction of opening.

The importance of vertex form cannot be overstated. It provides a powerful tool for analyzing and graphing quadratic functions, which is crucial in various fields, including physics, engineering, and economics. By expressing a quadratic function in vertex form, you can quickly identify the vertex, which is the minimum or maximum point of the parabola, depending on the direction of opening.

How Mathway Can Help with Vertex Form

Mathway is an online math problem solver that can be a valuable resource for anyone working with vertex form. With Mathway, you can input a quadratic function and instantly see it rewritten in vertex form. This can be a huge time-saver, especially when working with complex functions.

Mathway can also help you identify the vertex and other key features of the parabola, such as the axis of symmetry and direction of opening. By inputting the function into Mathway, you can quickly generate a graph of the parabola, which can be incredibly helpful for visualizing the function.

In addition to its graphing capabilities, Mathway also provides step-by-step solutions for converting quadratic functions to vertex form. This can be incredibly helpful for students or anyone looking to understand the process behind converting a function to vertex form.

Benefits of Using Mathway for Vertex Form

There are several benefits to using Mathway for vertex form, including:

• Time-saving: Mathway can instantly rewrite a quadratic function in vertex form, saving you time and effort.
• Accuracy: Mathway's algorithms ensure that the conversion is accurate, eliminating the risk of human error.
• Visualization: Mathway's graphing capabilities allow you to visualize the parabola, making it easier to understand key features.
• Step-by-step solutions: Mathway provides step-by-step solutions for converting quadratic functions to vertex form, helping you understand the process.

Working with Vertex Form in Mathway

Working with vertex form in Mathway is relatively straightforward. To convert a quadratic function to vertex form, simply input the function into Mathway's input field. Mathway will then rewrite the function in vertex form, providing you with the vertex and other key features of the parabola.

For example, let's say you want to convert the quadratic function f(x) = x^2 + 4x + 4 to vertex form. Simply input the function into Mathway, and it will rewrite it as f(x) = (x + 2)^2 - 4.

Step-by-Step Solution for Vertex Form

Here is a step-by-step solution for converting a quadratic function to vertex form using Mathway:

1. Input the quadratic function into Mathway's input field.
2. Click the "Enter" button to generate the solution.
3. Mathway will rewrite the function in vertex form, providing you with the vertex and other key features of the parabola.
4. Use the graphing capabilities to visualize the parabola and understand key features.

By following these steps, you can easily convert quadratic functions to vertex form using Mathway.

Practical Applications of Vertex Form

Vertex form has numerous practical applications in various fields, including physics, engineering, and economics. Here are a few examples:

• Projectile motion: Vertex form can be used to model the trajectory of projectiles, taking into account the initial velocity, angle of launch, and air resistance.
• Electrical circuits: Vertex form can be used to analyze the behavior of electrical circuits, including the voltage and current characteristics of the circuit.
• Economic modeling: Vertex form can be used to model economic systems, including the behavior of supply and demand curves.

Real-World Examples of Vertex Form

Here are a few real-world examples of vertex form in action:

• Google's search algorithm: Google's search algorithm uses a quadratic function to rank websites based on relevance. By converting this function to vertex form, Google can quickly identify the most relevant websites and return them to the user.
• GPS navigation: GPS navigation systems use quadratic functions to determine the shortest route between two points. By converting these functions to vertex form, GPS systems can quickly identify the optimal route and provide turn-by-turn directions.
• Medical imaging: Medical imaging techniques, such as MRI and CT scans, use quadratic functions to reconstruct images of the body. By converting these functions to vertex form, medical professionals can quickly identify tumors and other abnormalities.

Conclusion

In conclusion, vertex form is a powerful tool for analyzing and graphing quadratic functions. Mathway, a popular online math problem solver, can be a valuable resource for anyone working with vertex form. By using Mathway, you can quickly convert quadratic functions to vertex form, identify key features of the parabola, and visualize the function. With its numerous practical applications in various fields, vertex form is an essential concept for anyone working with quadratic functions.