Vertex Form Of A Quadratic Calculator Made Easy

Quick Links :

Unlocking the Power of Quadratic Calculations: Understanding Vertex Form

Quadratic equations are a fundamental concept in mathematics, and understanding their various forms is crucial for solving problems in algebra, geometry, and other fields. One of the most useful forms of a quadratic equation is the vertex form, which provides valuable insights into the graph of the quadratic function. In this article, we will delve into the world of vertex form, explore its benefits, and provide a comprehensive guide on how to use a vertex form of a quadratic calculator to simplify your calculations.

What is Vertex Form?

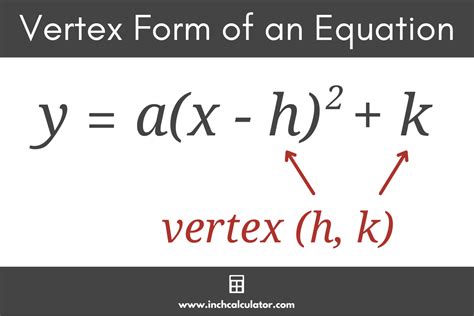
Vertex form is a way of expressing a quadratic equation in the form f(x) = a(x - h)^2 + k, where (h, k) represents the vertex of the parabola. This form is particularly useful because it allows us to easily identify the vertex, axis of symmetry, and the direction of the parabola's opening. By transforming a quadratic equation into vertex form, we can gain a deeper understanding of the graph's behavior and properties.

Why Use Vertex Form?

So, why is vertex form so important? Here are a few reasons why you should consider using vertex form in your quadratic calculations:

• Easy identification of the vertex: By expressing a quadratic equation in vertex form, we can quickly identify the vertex of the parabola, which is essential for graphing and analyzing the function.
• Simplified graphing: Vertex form makes it easy to graph a quadratic function by providing the vertex, axis of symmetry, and the direction of the parabola's opening.
• Improved problem-solving: Vertex form can help you solve problems involving quadratic functions more efficiently, especially when it comes to finding the maximum or minimum value of the function.

How to Convert to Vertex Form

Converting a quadratic equation to vertex form can be a bit tricky, but with the right steps, you can master this process. Here's a step-by-step guide on how to convert a quadratic equation to vertex form:

1. Start with the standard form: Begin with the standard form of a quadratic equation, which is ax^2 + bx + c = 0.
2. Complete the square: Complete the square by adding and subtracting (b/2a)^2 to the equation.
3. Factor the perfect square trinomial: Factor the perfect square trinomial to obtain the vertex form.

Example: Converting to Vertex Form

Suppose we want to convert the quadratic equation x^2 + 6x + 8 = 0 to vertex form. Here's how we can do it:

1. Complete the square: Add and subtract (b/2a)^2 = (6/2)^2 = 9 to the equation: x^2 + 6x + 9 - 9 + 8 = 0.
2. Factor the perfect square trinomial: Factor the perfect square trinomial: (x + 3)^2 - 1 = 0.
3. Write in vertex form: Write the equation in vertex form: f(x) = (x + 3)^2 - 1.

Using a Vertex Form of a Quadratic Calculator

While converting a quadratic equation to vertex form can be done manually, using a vertex form of a quadratic calculator can save you time and effort. A quadratic calculator can help you:

• Simplify calculations: A quadratic calculator can simplify the process of converting a quadratic equation to vertex form, reducing the risk of errors and saving you time.
• Visualize the graph: Many quadratic calculators come with graphing capabilities, allowing you to visualize the graph of the quadratic function and gain a deeper understanding of its behavior.

Benefits of Using a Quadratic Calculator

Here are some benefits of using a quadratic calculator:

• Improved accuracy: A quadratic calculator can reduce the risk of errors and provide accurate results, giving you confidence in your calculations.
• Increased efficiency: A quadratic calculator can save you time and effort, allowing you to focus on more complex problems and tasks.
• Enhanced understanding: By visualizing the graph of the quadratic function, you can gain a deeper understanding of its behavior and properties.

Conclusion: Mastering Vertex Form with a Quadratic Calculator

Mastering vertex form is an essential skill for anyone working with quadratic equations. By understanding the benefits of vertex form and using a vertex form of a quadratic calculator, you can simplify your calculations, improve your accuracy, and gain a deeper understanding of the graph's behavior. Whether you're a student, teacher, or professional, a quadratic calculator can be a valuable tool in your mathematical toolkit.

Now, it's your turn to try using a vertex form of a quadratic calculator. Share your experiences, tips, and tricks in the comments below, and don't forget to share this article with your friends and colleagues who may benefit from mastering vertex form.