Vertex form is a powerful tool in calculus that allows you to analyze and understand the behavior of functions in a more intuitive way. By mastering vertex form, you'll be able to tackle complex problems with ease and precision. In this article, we'll explore five ways to master vertex form in calculus, along with practical examples and expert tips to help you along the way.
Understanding the Basics of Vertex Form
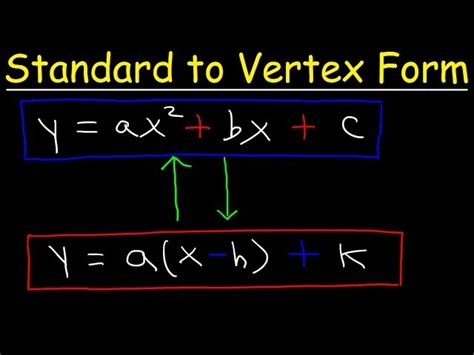
Vertex form is a way of expressing a quadratic function in the form f(x) = a(x - h)^2 + k, where (h, k) is the vertex of the parabola. This form is particularly useful for analyzing the maximum or minimum value of a function, as well as the axis of symmetry.

1. Learn to Identify the Vertex
The first step to mastering vertex form is to learn how to identify the vertex of a parabola. The vertex is the point where the parabola changes direction, and it's the minimum or maximum value of the function. To find the vertex, you can use the formula x = -b / 2a, where a and b are coefficients of the quadratic equation.
For example, consider the quadratic function f(x) = x^2 + 4x + 4. To find the vertex, we can use the formula x = -b / 2a = -4 / 2(1) = -2. Plugging this value back into the function, we get f(-2) = (-2)^2 + 4(-2) + 4 = 4 - 8 + 4 = 0. Therefore, the vertex of the parabola is (-2, 0).
**2. Practice Converting to Vertex Form**
Converting a quadratic function to vertex form is a crucial skill to master. To do this, you'll need to complete the square, which involves rewriting the quadratic expression in a perfect square trinomial form.
For example, consider the quadratic function f(x) = x^2 + 6x + 8. To convert this to vertex form, we can complete the square by adding and subtracting (b/2)^2 = (6/2)^2 = 9 inside the parentheses: f(x) = x^2 + 6x + 9 - 9 + 8 = (x + 3)^2 - 1.

3. Understand the Relationship Between Vertex Form and the Graph
Understanding the relationship between vertex form and the graph of a quadratic function is crucial for mastering vertex form. The vertex form tells you the minimum or maximum value of the function, as well as the axis of symmetry.
For example, consider the quadratic function f(x) = (x - 2)^2 + 3. From the vertex form, we can see that the vertex of the parabola is (2, 3), and the axis of symmetry is x = 2.
**The Graph of a Quadratic Function in Vertex Form**
- The graph opens upwards if a > 0
- The graph opens downwards if a < 0
- The vertex is the minimum or maximum value of the function
- The axis of symmetry is x = h

4. Learn to Analyze the Behavior of the Function
Mastering vertex form also involves learning to analyze the behavior of the function. This includes identifying the maximum or minimum value, as well as the axis of symmetry.
For example, consider the quadratic function f(x) = -(x - 1)^2 + 2. From the vertex form, we can see that the vertex of the parabola is (1, 2), and the axis of symmetry is x = 1. We can also see that the graph opens downwards, indicating a maximum value.
**5. Practice, Practice, Practice!**
Finally, the key to mastering vertex form is to practice, practice, practice! Work through numerous examples and exercises to reinforce your understanding of vertex form.
Some practice exercises to try:
- Convert the quadratic function f(x) = x^2 + 2x + 1 to vertex form
- Find the vertex of the parabola f(x) = x^2 - 4x + 4
- Identify the axis of symmetry of the graph f(x) = (x - 1)^2 + 2

Take Your Calculus Skills to the Next Level
Mastering vertex form is an essential skill for any calculus student. By following these five tips, you'll be well on your way to becoming a vertex form expert. Remember to practice regularly, and don't be afraid to ask for help if you get stuck.
Share your own tips and strategies for mastering vertex form in the comments below! And if you have any questions or need further clarification, don't hesitate to ask.
FAQs
What is vertex form in calculus?
+Vertex form is a way of expressing a quadratic function in the form f(x) = a(x - h)^2 + k, where (h, k) is the vertex of the parabola.
How do I convert a quadratic function to vertex form?
+To convert a quadratic function to vertex form, you'll need to complete the square by adding and subtracting (b/2)^2 inside the parentheses.
What is the relationship between vertex form and the graph of a quadratic function?
+The vertex form tells you the minimum or maximum value of the function, as well as the axis of symmetry. The graph opens upwards if a > 0 and downwards if a < 0.