Convert Vertex And Point To Standard Form Easily

Quick Links :

Converting vertex and point forms to standard form is an essential skill in mathematics, particularly in algebra and geometry. The standard form of a linear equation is Ax + By = C, where A, B, and C are integers, and A is non-negative. In this article, we will explore the steps and methods to convert vertex and point forms to standard form easily.

Understanding Vertex and Point Forms

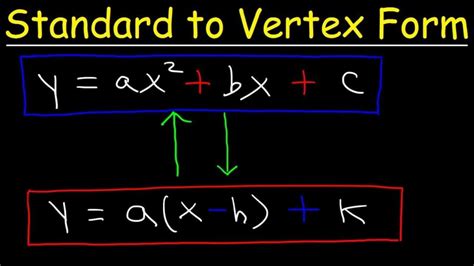
Before we dive into the conversion process, let's first understand what vertex and point forms are. The vertex form of a linear equation is y = a(x - h) + k, where (h, k) is the vertex of the line. The point form, on the other hand, is y - y1 = m(x - x1), where (x1, y1) is a point on the line, and m is the slope.

Why Convert to Standard Form?

Converting vertex and point forms to standard form is essential for several reasons:

• Simplifies equations: Standard form makes it easier to compare and solve equations.
• Eases graphing: Standard form allows us to graph lines quickly and accurately.
• Enhances problem-solving: Standard form enables us to solve systems of equations and inequalities more efficiently.

Converting Vertex Form to Standard Form

To convert vertex form to standard form, follow these steps:

1. Multiply the terms inside the parentheses by the coefficient a.
2. Distribute the terms to simplify the equation.
3. Move all terms to one side of the equation to set it equal to zero.
4. Combine like terms to simplify further.

Example:

Vertex form: y = 2(x - 3) + 4 Standard form: 2x - y = -2

Converting Point Form to Standard Form

To convert point form to standard form, follow these steps:

1. Multiply the terms inside the parentheses by the slope m.
2. Distribute the terms to simplify the equation.
3. Move all terms to one side of the equation to set it equal to zero.
4. Combine like terms to simplify further.

Example:

Point form: y - 2 = 3(x - 4) Standard form: 3x - y = -10

Tips and Tricks

• When converting vertex form to standard form, ensure that the coefficient of x is non-negative.
• When converting point form to standard form, ensure that the slope is non-zero.
• Use online tools or calculators to check your work and verify the conversion.

Common Mistakes to Avoid

• Forgetting to distribute terms inside the parentheses.
• Failing to combine like terms.
• Not checking the coefficient of x in standard form.

Conclusion and Next Steps

Converting vertex and point forms to standard form is a valuable skill that can simplify equations, ease graphing, and enhance problem-solving. By following the steps and methods outlined in this article, you can easily convert vertex and point forms to standard form. Practice makes perfect, so be sure to work through examples and exercises to reinforce your understanding.