Standard Form Worksheet With Answers

Quick Links :

The concept of standard form is a fundamental idea in mathematics, particularly in algebra and geometry. It provides a convenient way to express numbers, equations, and functions in a unique and simplified form. In this article, we will delve into the world of standard form, exploring its definition, importance, and applications. We will also provide a comprehensive worksheet with answers to help you practice and reinforce your understanding of this essential concept.

What is Standard Form?

Standard form is a way of expressing numbers, equations, and functions in a specific format. In mathematics, standard form is used to simplify complex expressions and make them easier to work with. For example, the standard form of a linear equation is ax + by = c, where a, b, and c are constants.

Importance of Standard Form

Standard form is essential in mathematics because it provides a common language for expressing and solving equations. It helps to:

• Simplify complex expressions
• Identify patterns and relationships
• Solve equations and inequalities
• Graph functions and equations

Types of Standard Form

There are several types of standard form, including:

• Standard Form of a Linear Equation: ax + by = c
• Standard Form of a Quadratic Equation: ax^2 + bx + c = 0
• Standard Form of a Polynomial: ax^n + bx^(n-1) +... + cx + d = 0

Converting to Standard Form

Converting an equation or expression to standard form involves simplifying and rearranging the terms. Here are some steps to follow:

1. Simplify the expression by combining like terms.
2. Rearrange the terms to put the variable on one side of the equation.
3. Move all constants to the other side of the equation.

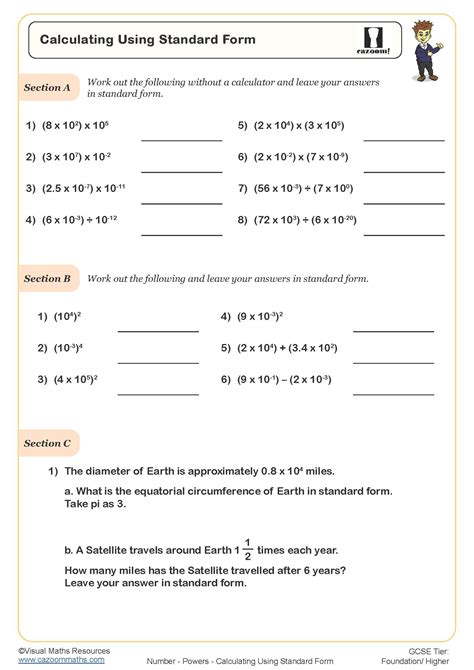
Standard Form Worksheet

Now it's time to practice! Here is a comprehensive worksheet with answers to help you master the concept of standard form.

Part 1: Converting to Standard Form

1. Convert the equation 2x + 5 = 11 to standard form. Answer: 2x - 5 = 6
2. Convert the equation x - 3 = 7 to standard form. Answer: x = 10
3. Convert the equation 4x + 2 = 16 to standard form. Answer: 4x - 2 = 14

Part 2: Identifying Standard Form

1. Identify the standard form of the equation 3x + 2y = 5. Answer: Standard Form of a Linear Equation
2. Identify the standard form of the equation x^2 + 4x + 4 = 0. Answer: Standard Form of a Quadratic Equation
3. Identify the standard form of the equation 2x^3 - 5x^2 + 3x - 1 = 0. Answer: Standard Form of a Polynomial

Part 3: Solving Equations in Standard Form

1. Solve the equation 2x + 3 = 7 in standard form. Answer: x = 2
2. Solve the equation x - 2 = 9 in standard form. Answer: x = 11
3. Solve the equation 4x - 2 = 14 in standard form. Answer: x = 4

Conclusion

In conclusion, standard form is an essential concept in mathematics that provides a convenient way to express numbers, equations, and functions in a unique and simplified form. By mastering the concept of standard form, you will be able to simplify complex expressions, identify patterns and relationships, and solve equations and inequalities with ease.