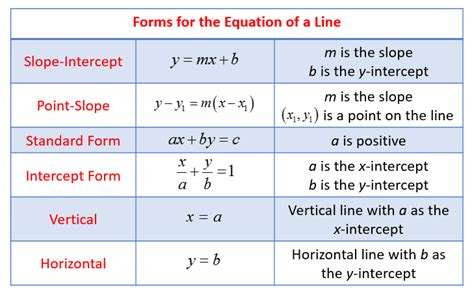
Lines with no slope, also known as horizontal lines, are a fundamental concept in mathematics and geometry. They play a crucial role in various mathematical operations, including graphing, algebra, and calculus. In this article, we will delve into the world of horizontal lines, exploring their standard form, equations, and graphing.
The Importance of Understanding Horizontal Lines
Horizontal lines are essential in mathematics as they help us understand and visualize relationships between variables. They are used to represent constant values, boundaries, and thresholds in various mathematical models. Moreover, horizontal lines are used in real-world applications, such as architecture, engineering, and design, to create symmetries, boundaries, and patterns.
What is the Standard Form of a Horizontal Line?

The standard form of a horizontal line is y = c, where c is a constant value. This equation represents a line that is parallel to the x-axis and intersects the y-axis at the point (0, c). The standard form of a horizontal line is unique in that it has no slope, as the y-coordinate remains constant for all values of x.
Equations of Horizontal Lines
Horizontal lines can be represented by various equations, including:
- y = c, where c is a constant value
- x = c, where c is a constant value (vertical line)
- ax + by = c, where a and b are coefficients and c is a constant value (general form)
However, the standard form of a horizontal line is y = c, which provides a clear and concise representation of the line's equation.
Graphing Horizontal Lines

Graphing horizontal lines is a straightforward process. To graph a horizontal line, follow these steps:
- Identify the equation of the line in standard form (y = c).
- Plot the point (0, c) on the coordinate plane.
- Draw a horizontal line through the point (0, c), extending it infinitely in both directions.
The resulting graph will be a horizontal line that intersects the y-axis at the point (0, c).
Examples of Horizontal Lines
Here are a few examples of horizontal lines and their equations:
- y = 2 (horizontal line that intersects the y-axis at the point (0, 2))
- y = -3 (horizontal line that intersects the y-axis at the point (0, -3))
- y = 0 (x-axis, which is a horizontal line that intersects the y-axis at the point (0, 0))
These examples illustrate the standard form of horizontal lines and demonstrate how to graph them on the coordinate plane.
Real-World Applications of Horizontal Lines

Horizontal lines have numerous real-world applications, including:
- Architecture: Horizontal lines are used to create symmetries, boundaries, and patterns in building designs.
- Engineering: Horizontal lines are used to represent constant values, such as water levels, in engineering designs.
- Design: Horizontal lines are used to create visual balance and harmony in graphic designs.
These applications demonstrate the importance of horizontal lines in various fields and highlight their role in creating symmetries, boundaries, and patterns.
Conclusion
In conclusion, horizontal lines are a fundamental concept in mathematics and geometry. Their standard form, equations, and graphing are essential in understanding and visualizing relationships between variables. Real-world applications of horizontal lines include architecture, engineering, and design, where they are used to create symmetries, boundaries, and patterns.
We hope this article has provided you with a comprehensive understanding of horizontal lines and their importance in mathematics and real-world applications. Share your thoughts and experiences with horizontal lines in the comments below, and don't forget to share this article with your friends and colleagues.
What is the standard form of a horizontal line?
+The standard form of a horizontal line is y = c, where c is a constant value.
How do you graph a horizontal line?
+To graph a horizontal line, plot the point (0, c) on the coordinate plane and draw a horizontal line through the point, extending it infinitely in both directions.
What are some real-world applications of horizontal lines?
+Horizontal lines have numerous real-world applications, including architecture, engineering, and design, where they are used to create symmetries, boundaries, and patterns.