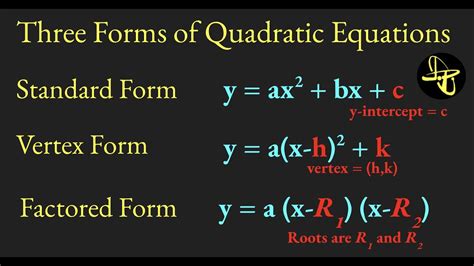
Quadratic equations are a fundamental concept in mathematics, and they have numerous applications in various fields, including physics, engineering, and computer science. A quadratic equation is a polynomial equation of degree two, which means the highest power of the variable is two. In this article, we will explore the three main forms of quadratic equations, their characteristics, and provide examples to illustrate each form.
What are Quadratic Equations?

Quadratic equations are equations of the form ax^2 + bx + c = 0, where a, b, and c are constants, and x is the variable. The solutions to quadratic equations can be real or complex numbers. Quadratic equations can be solved using various methods, including factoring, quadratic formula, and graphing.
Standard Form of Quadratic Equations

The standard form of a quadratic equation is ax^2 + bx + c = 0, where a, b, and c are constants, and a ≠ 0. This form is also known as the general form of a quadratic equation.
Characteristics of Standard Form Quadratic Equations
- The coefficient of the x^2 term (a) is non-zero.
- The coefficients of the x term (b) and the constant term (c) can be any real numbers.
- The solutions to the equation can be real or complex numbers.
Example: x^2 + 4x + 4 = 0 is a standard form quadratic equation.
Vertex Form of Quadratic Equations

The vertex form of a quadratic equation is a(x - h)^2 + k = 0, where (h, k) is the vertex of the parabola. This form is also known as the vertex form of a quadratic function.
Characteristics of Vertex Form Quadratic Equations
- The equation is written in the form a(x - h)^2 + k = 0, where (h, k) is the vertex of the parabola.
- The coefficient of the squared term (a) can be any real number.
- The solutions to the equation can be real or complex numbers.
Example: (x - 2)^2 - 3 = 0 is a vertex form quadratic equation.
Factored Form of Quadratic Equations

The factored form of a quadratic equation is (x - r)(x - s) = 0, where r and s are the roots of the equation. This form is also known as the factored form of a quadratic function.
Characteristics of Factored Form Quadratic Equations
- The equation is written in the form (x - r)(x - s) = 0, where r and s are the roots of the equation.
- The coefficients of the linear terms (x - r) and (x - s) can be any real numbers.
- The solutions to the equation are the roots of the equation, which can be real or complex numbers.
Example: (x - 2)(x + 3) = 0 is a factored form quadratic equation.
Now that we have explored the three main forms of quadratic equations, let's summarize the key points:
- Standard form: ax^2 + bx + c = 0
- Vertex form: a(x - h)^2 + k = 0
- Factored form: (x - r)(x - s) = 0
Each form has its own characteristics and advantages. The standard form is the most common form of a quadratic equation, while the vertex form is useful for graphing and finding the vertex of a parabola. The factored form is useful for finding the roots of a quadratic equation.
We hope this article has helped you understand the three main forms of quadratic equations. Whether you are a student, teacher, or simply a math enthusiast, quadratic equations are an essential concept to grasp.
What is the difference between a quadratic equation and a quadratic function?
+A quadratic equation is an equation of the form ax^2 + bx + c = 0, while a quadratic function is a function of the form f(x) = ax^2 + bx + c.
How do I solve a quadratic equation?
+There are several methods to solve a quadratic equation, including factoring, quadratic formula, and graphing.
What is the vertex form of a quadratic equation?
+The vertex form of a quadratic equation is a(x - h)^2 + k = 0, where (h, k) is the vertex of the parabola.
We hope you found this article informative and helpful. If you have any questions or comments, please feel free to ask.