Converting Standard Form To Slope Intercept Form Made Easy

Quick Links :

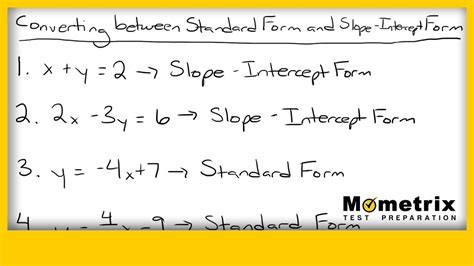
Understanding the intricacies of linear equations is a fundamental aspect of mathematics, particularly in algebra and geometry. Two of the most common forms of linear equations are the Standard Form and the Slope-Intercept Form. While both forms represent the same line, they differ in structure and usage. Converting from one form to another is a crucial skill that allows for a deeper understanding and manipulation of linear equations. In this article, we will delve into the process of converting Standard Form to Slope-Intercept Form, making it easy and accessible for readers of all levels.

Understanding Standard Form

Standard Form, also known as General Form, is a way of expressing linear equations in the form of Ax + By = C, where A, B, and C are integers, and A and B are not both zero. This form is useful for various algebraic manipulations and for finding the x and y intercepts of a line. However, it does not immediately reveal the slope or the y-intercept of the line, making it less intuitive for graphing and analyzing the behavior of the line.

Example of Standard Form

Consider the equation 2x + 3y = 12. This is a typical example of a linear equation in Standard Form, where A = 2, B = 3, and C = 12.

Understanding Slope-Intercept Form

Slope-Intercept Form, on the other hand, is a linear equation in the form of y = mx + b, where m is the slope of the line and b is the y-intercept. This form is particularly useful for graphing lines, as it immediately reveals the slope and the y-intercept, allowing for quick identification of the line's orientation and position on the coordinate plane.

Example of Slope-Intercept Form

Consider the equation y = 2x - 3. Here, m = 2 (slope) and b = -3 (y-intercept).

Converting Standard Form to Slope-Intercept Form

To convert a linear equation from Standard Form to Slope-Intercept Form, follow these steps:

1. Isolate the variable y: Rearrange the equation so that all terms involving y are on one side of the equation and everything else is on the other side. This usually involves moving the x term to the other side.

2. Factor out the coefficient of y: If the coefficient of y is not 1, divide every term in the equation by this coefficient. This will ensure that the coefficient of y is 1, which is required for the Slope-Intercept Form.

3. Identify the slope and y-intercept: Once the equation is in the form y = mx + b, the coefficient of x (m) is the slope, and the constant term (b) is the y-intercept.

Example Conversion

Let's convert the Standard Form equation 2x + 3y = 12 to Slope-Intercept Form:

1. Isolate the variable y: 3y = -2x + 12

2. Factor out the coefficient of y: y = (-2/3)x + 4

In this Slope-Intercept Form, the slope (m) is -2/3, and the y-intercept (b) is 4.

Benefits of Converting to Slope-Intercept Form

Converting Standard Form to Slope-Intercept Form offers several benefits, including:

• Easier graphing: The Slope-Intercept Form immediately reveals the slope and y-intercept, making it easier to graph the line.

• Quick analysis: Understanding the slope and y-intercept allows for quick analysis of the line's behavior and orientation on the coordinate plane.

• Simpler equation manipulation: Many algebraic manipulations and calculations are simplified when working with the Slope-Intercept Form.

Conclusion - Mastering the Conversion

Mastering the conversion from Standard Form to Slope-Intercept Form is an essential skill in mathematics, particularly in algebra and geometry. By following the simple steps outlined in this article, readers can easily convert linear equations, gaining a deeper understanding and improving their ability to graph, analyze, and manipulate lines. Whether you're a student, teacher, or simply a math enthusiast, converting Standard Form to Slope-Intercept Form is a valuable tool to have in your mathematical toolkit.

We hope this article has been informative and helpful. If you have any questions or need further clarification on any of the topics discussed, please feel free to ask in the comments section below. Don't forget to share this article with friends and colleagues who might find it useful!