Mastering Ellipse Equations: 5 Key Standard Forms

Quick Links :

Ellipse equations are a fundamental concept in mathematics, particularly in algebra and geometry. These equations describe the shape and size of an ellipse, a closed curve where the sum of the distances from two fixed points (the foci) is constant. Mastering ellipse equations is essential for various fields, including physics, engineering, and architecture. In this article, we will delve into the world of ellipse equations, exploring the five key standard forms and providing examples, formulas, and practical applications.

Understanding Ellipse Equations

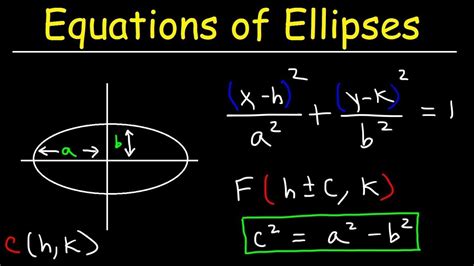
An ellipse equation is a mathematical representation of an ellipse in a two-dimensional plane. It is defined by its major and minor axes, which are perpendicular to each other. The major axis is the longest diameter, while the minor axis is the shortest diameter. The foci of an ellipse are the two fixed points inside the ellipse that help define its shape.

The Importance of Ellipse Equations

Ellipse equations have numerous practical applications in various fields, including:

• Physics: Ellipse equations are used to describe the orbits of planets, comets, and other celestial bodies.
• Engineering: Ellipse equations are used in the design of bridges, tunnels, and other structures.
• Architecture: Ellipse equations are used in the design of buildings, stadiums, and other structures.

Standard Form 1: Horizontal Major Axis

The first standard form of an ellipse equation is when the major axis is horizontal. The equation is:

(x - h)^2/a^2 + (y - k)^2/b^2 = 1

where (h, k) is the center of the ellipse, a is the length of the semi-major axis, and b is the length of the semi-minor axis.

Example

Find the equation of an ellipse with a center at (2, 3), a semi-major axis of 4, and a semi-minor axis of 2.

Solution: (x - 2)^2/4^2 + (y - 3)^2/2^2 = 1

Standard Form 2: Vertical Major Axis

The second standard form of an ellipse equation is when the major axis is vertical. The equation is:

(x - h)^2/b^2 + (y - k)^2/a^2 = 1

where (h, k) is the center of the ellipse, a is the length of the semi-major axis, and b is the length of the semi-minor axis.

Example

Find the equation of an ellipse with a center at (1, 2), a semi-major axis of 3, and a semi-minor axis of 1.

Solution: (x - 1)^2/1^2 + (y - 2)^2/3^2 = 1

Standard Form 3: Center at the Origin

The third standard form of an ellipse equation is when the center of the ellipse is at the origin (0, 0). The equation is:

x^2/a^2 + y^2/b^2 = 1

where a is the length of the semi-major axis and b is the length of the semi-minor axis.

Example

Find the equation of an ellipse with a semi-major axis of 5 and a semi-minor axis of 3.

Solution: x^2/5^2 + y^2/3^2 = 1

Standard Form 4: Horizontal Major Axis with Center at the Origin

The fourth standard form of an ellipse equation is when the major axis is horizontal and the center is at the origin. The equation is:

x^2/a^2 + y^2/b^2 = 1

where a is the length of the semi-major axis and b is the length of the semi-minor axis.

Example

Find the equation of an ellipse with a semi-major axis of 4 and a semi-minor axis of 2.

Solution: x^2/4^2 + y^2/2^2 = 1

Standard Form 5: Vertical Major Axis with Center at the Origin

The fifth standard form of an ellipse equation is when the major axis is vertical and the center is at the origin. The equation is:

x^2/b^2 + y^2/a^2 = 1

where a is the length of the semi-major axis and b is the length of the semi-minor axis.

Example

Find the equation of an ellipse with a semi-major axis of 3 and a semi-minor axis of 1.

Solution: x^2/1^2 + y^2/3^2 = 1

Now that we have explored the five key standard forms of ellipse equations, it's time to put your knowledge to the test. Try solving some practice problems to reinforce your understanding of ellipse equations.

Conclusion

Ellipse equations are a fundamental concept in mathematics, and mastering them is essential for various fields. In this article, we have explored the five key standard forms of ellipse equations, providing examples, formulas, and practical applications. By understanding these standard forms, you can solve a wide range of problems involving ellipses.