Are you tired of dealing with exponents and wanting to convert them to radicals? Look no further! In this article, we will walk you through the process of converting exponents to radicals in 3 easy steps. Whether you're a student, teacher, or simply a math enthusiast, this guide is perfect for anyone looking to master this fundamental concept in mathematics.
What are Exponents and Radicals?

Before we dive into the conversion process, let's quickly review what exponents and radicals are. Exponents are shorthand for repeated multiplication, where a base number is raised to a power. For example, 2^3 represents 2 multiplied by itself three times (2 × 2 × 2). Radicals, on the other hand, represent the opposite operation, where a number is taken to a fractional power. For instance, the square root of 16 (√16) represents the number that, when multiplied by itself, equals 16.
Step 1: Understand the Relationship Between Exponents and Radicals

The first step in converting exponents to radicals is to understand the relationship between the two. In general, when you have an exponent with a fractional power, you can convert it to a radical using the following rule:
a^(1/n) = nth root of a
In other words, if you have an exponent with a power of 1/n, you can rewrite it as the nth root of the base number. For example, 2^(1/3) can be rewritten as the cube root of 2 (∛2).
Examples of Converting Exponents to Radicals
- 2^(1/2) = √2 (square root of 2)
- 3^(1/3) = ∛3 (cube root of 3)
- 4^(1/4) = ∜4 (fourth root of 4)
Step 2: Apply the Rule to Convert Exponents to Radicals

Now that you understand the relationship between exponents and radicals, you can apply the rule to convert exponents to radicals. Here are some examples:
- 2^3 = (2^3)^(1/2) = (√2)^3 (converting 2^3 to a radical using the rule)
- 3^4 = (3^4)^(1/4) = ∜3^4 (converting 3^4 to a radical using the rule)
- 5^2 = (5^2)^(1/2) = √5^2 (converting 5^2 to a radical using the rule)
Tips and Tricks for Converting Exponents to Radicals
- Make sure to simplify the radical expression by combining like terms.
- Use the rule a^(1/n) = nth root of a to convert exponents to radicals.
- Be careful when dealing with negative exponents, as they may require additional steps to convert to radicals.
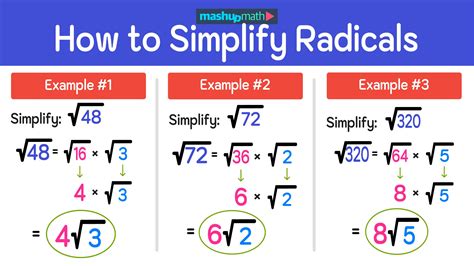
Step 3: Simplify the Radical Expression

The final step in converting exponents to radicals is to simplify the radical expression. This may involve combining like terms, factoring out perfect squares or cubes, and canceling out any common factors.
Examples of Simplifying Radical Expressions
- √2^2 = √(2^2) = √4 = 2 (simplifying the radical expression)
- ∛3^3 = ∛(3^3) = ∛27 = 3 (simplifying the radical expression)
- ∜4^4 = ∜(4^4) = ∜256 = 4 (simplifying the radical expression)
By following these three easy steps, you can convert exponents to radicals with confidence. Remember to understand the relationship between exponents and radicals, apply the rule to convert exponents to radicals, and simplify the radical expression. With practice and patience, you'll become a pro at converting exponents to radicals in no time!
What do you think? Do you have any questions or tips for converting exponents to radicals? Share your thoughts in the comments below!
What is the relationship between exponents and radicals?
+The relationship between exponents and radicals is that a fractional exponent can be rewritten as a radical using the rule a^(1/n) = nth root of a.
How do I convert exponents to radicals?
+To convert exponents to radicals, follow these steps: understand the relationship between exponents and radicals, apply the rule a^(1/n) = nth root of a, and simplify the radical expression.
What are some common mistakes to avoid when converting exponents to radicals?
+Some common mistakes to avoid when converting exponents to radicals include not simplifying the radical expression, forgetting to combine like terms, and not canceling out common factors.