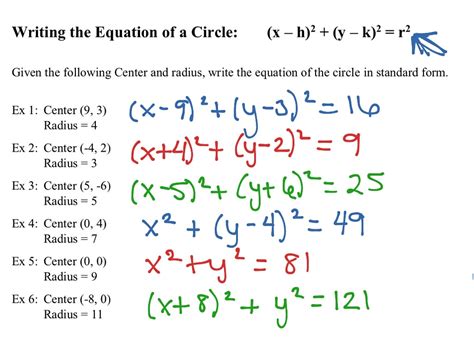
Circle Equation Made Easy: 5 Steps To Standard Form

Quick Links :

Circle equations can be a daunting topic for many students, but with the right approach, they can be made easy to understand and work with. In this article, we'll break down the process of converting a circle equation into standard form in 5 simple steps. By the end of this article, you'll be able to confidently convert any circle equation into standard form.

A circle equation is a mathematical representation of a circle in a Cartesian coordinate system. It's used to define the center, radius, and other properties of a circle. The standard form of a circle equation is (x - h)^2 + (y - k)^2 = r^2, where (h, k) is the center of the circle, and r is the radius.

Why is Standard Form Important?

Standard form is essential in mathematics and real-life applications, such as engineering, physics, and architecture. It provides a clear and concise way to represent circles and makes it easier to perform calculations and transformations. By converting a circle equation into standard form, you can:

• Identify the center and radius of the circle
• Calculate the area and circumference of the circle
• Perform translations, rotations, and reflections on the circle
• Solve problems involving circles in various fields

Benefits of Standard Form

Some of the benefits of standard form include:

• Simplified calculations: Standard form makes it easier to perform calculations, such as finding the area and circumference of a circle.
• Easy identification: Standard form allows you to easily identify the center and radius of a circle.
• Versatility: Standard form can be used in various mathematical and real-life applications.

5 Steps to Standard Form

Now that we've discussed the importance of standard form, let's dive into the 5 steps to convert a circle equation into standard form.

Step 1: Expand the Equation

The first step is to expand the given equation. This involves multiplying out any brackets and combining like terms.

Example: (x + 3)^2 + (y - 2)^2 = 16

Expanded equation: x^2 + 6x + 9 + y^2 - 4y + 4 = 16

Step 2: Move the Constant Term

The next step is to move the constant term to the right-hand side of the equation.

Example: x^2 + 6x + 9 + y^2 - 4y + 4 = 16

Moved constant term: x^2 + 6x + y^2 - 4y = 16 - 9 - 4

Step 3: Complete the Square

The third step is to complete the square for both the x and y terms. This involves adding and subtracting the square of half the coefficient of x and y.

Example: x^2 + 6x + y^2 - 4y = 3

Completed square: x^2 + 6x + 9 + y^2 - 4y + 4 = 3 + 9 + 4

Step 4: Factorize the Equation

The fourth step is to factorize the equation into the standard form.

Example: x^2 + 6x + 9 + y^2 - 4y + 4 = 16

Factorized equation: (x + 3)^2 + (y - 2)^2 = 16

Step 5: Write the Standard Form

The final step is to write the equation in standard form.

Example: (x + 3)^2 + (y - 2)^2 = 16

Standard form: (x - (-3))^2 + (y - 2)^2 = 4^2

Common Mistakes to Avoid

When converting a circle equation into standard form, there are some common mistakes to avoid:

• Not expanding the equation correctly
• Not moving the constant term to the right-hand side
• Not completing the square correctly
• Not factorizing the equation correctly
• Not writing the equation in standard form

By avoiding these common mistakes, you can ensure that you convert the circle equation into standard form correctly.

Conclusion and Next Steps

In this article, we've discussed the importance of standard form and the 5 steps to convert a circle equation into standard form. By following these steps, you can confidently convert any circle equation into standard form.

To take your skills to the next level, practice converting different types of circle equations into standard form. You can also explore other mathematical topics, such as ellipse equations and parabola equations.

Share your thoughts and feedback in the comments section below. What do you find challenging about converting circle equations into standard form? How do you think you can apply this knowledge in real-life situations?